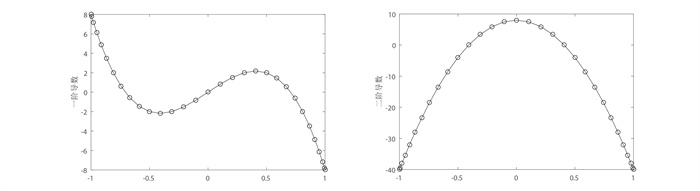
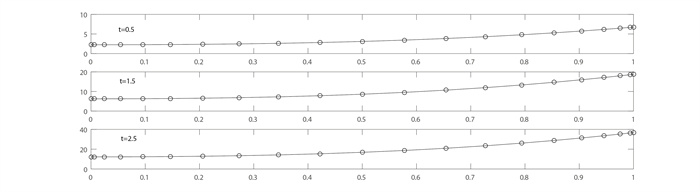
Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (5): 1496-1505.
Previous Articles Next Articles
A High Order MQ Quasi-Interpolation Method for Time Fractional Black-Scholes Model
Shengliang Zhang*( ),Junxian Huang
),Junxian Huang
- Department of Applied Economics, College of Economics and Management, Nanjing Forestry University, Nanjing 210037
-
Received:2021-09-08Online:2022-10-26Published:2022-09-30 -
Contact:Shengliang Zhang E-mail:10110180035@fudan.edu.cn -
Supported by:the(21YJC790162);the Jiangsu Provincial Social Science Foundation(22EYBo10)
CLC Number:
- O174
Cite this article
Shengliang Zhang,Junxian Huang. A High Order MQ Quasi-Interpolation Method for Time Fractional Black-Scholes Model[J].Acta mathematica scientia,Series A, 2022, 42(5): 1496-1505.
share this article
"
| α | M | L2 | rate (L2) | L∞ | rate (L∞) |
| 0.7 | 4 | 6.732 × 10?3 | 7.821 × 10?3 | ||
| 8 | 1.587 × 10?3 | 2.09 | 1.784 × 10?3 | 2.13 | |
| 16 | 2.986 × 10?4 | 2.41 | 3.366 × 10?4 | 2.42 | |
| 32 | 3.867 × 10?5 | 2.95 | 4.586 × 10?5 | 2.87 | |
| 64 | 3.225 × 10?6 | 3.58 | 4.133 × 10?6 | 3.47 | |
| 0.3 | 4 | 6.851 × 10?3 | 8.070 × 10?3 | ||
| 8 | 1.613 × 10?3 | 2.09 | 1.882 × 10?3 | 2.10 | |
| 16 | 3.055 × 10?4 | 2.40 | 3.541 × 10?4 | 2.41 | |
| 32 | 4.012 × 10?5 | 2.93 | 4.630 × 10?5 | 2.93 | |
| 64 | 3.396 × 10?6 | 3.56 | 3.895 × 10?6 | 3.57 |
"
| α | N | L2 | rate (L2) | L∞ | rate (L∞) |
| 0.7 | 10 | 3.124 × 10?3 | 4.301 × 10?3 | ||
| 20 | 1.351 × 10?3 | 1.27 | 1.847 × 10?3 | 1.22 | |
| 40 | 5.240 × 10?4 | 1.28 | 7.403 × 10?4 | 1.31 | |
| 80 | 2.142 × 10?4 | 1.32 | 2.854 × 10?4 | 1.37 | |
| 160 | 8.096 × 10?5 | 1.33 | 1.009 × 10?4 | 1.48 | |
| 0.3 | 10 | 3.670 × 10?3 | 4.984 × 10?3 | ||
| 20 | 1.142 × 10?3 | 1.68 | 1.583 × 10?3 | 1.66 | |
| 40 | 3.560 × 10?4 | 1.69 | 4.871 × 10?4 | 1.69 | |
| 80 | 1.084 × 10?4 | 1.71 | 1.494 × 10?4 | 1.71 | |
| 160 | 3.330 × 10?5 | 1.74 | 4.447 × 10?5 | 1.74 |
"
| c | α = 0.7 | α = 0.3 | ||
| L2 | L∞ | L2 | L∞ | |
| 0.1 | 5.987 × 10?5 | 8.344 × 10?5 | 6.783 × 10?5 | 9.045 × 10?5 |
| 0.2 | 3.867 × 10?5 | 4.586 × 10?5 | 4.012 × 10?5 | 4.630 × 10?5 |
| 0.3 | 4.637 × 10?5 | 6.690 × 10?5 | 6.532 × 10?5 | 8.046 × 10?5 |
| 0.4 | 5.450 × 10?5 | 7.098 × 10?5 | 6.998 × 10?5 | 8.993 × 10?5 |
| 0.5 | 6.721 × 10?5 | 9.873 × 10?5 | 7.356 × 10?5 | 9.567 × 10?5 |
| 0.6 | 7.983 × 10?5 | 1.032 × 10?4 | 8.220 × 10?5 | 1.356 × 10?4 |
"
| c | α=0.7 | α=0.3 | ||
| L2 | L∞ | L2 | L∞ | |
| 0.73 | 9.332 × 10?5 | 1.437 × 10?4 | 1.230 × 10?4 | 3.877 × 10?4 |
| 0.63 | 2.173 × 10?5 | 3.245 × 10?5 | 3.875 × 10?5 | 4.690 × 10?5 |
| 0.53 | 2.356 × 10?4 | 3.678 × 10?4 | 3.256 × 10?4 | 4.730 × 10?4 |
| 0.43 | 5.889 × 10?4 | 7.356 × 10?4 | 6.709 × 10?4 | 9.034 × 10?4 |
| 0.33 | 1.853 × 10?3 | 2.801 × 10?3 | 2.089 × 10?3 | 3.903 × 10?3 |
| 0.23 | 4.743 × 10?2 | 6.082 × 10?2 | 6.044 × 10?2 | 9.871 × 10?2 |
| 1 |
Beatson R K , Dyn N . Multiquadric B-Splines. J Approx Theory, 1996, 87 (1): 1- 24
doi: 10.1006/jath.1996.0089 |
| 2 |
Black F , Scholes M . The pricing of options and corporate liabilities. J Polit Econ, 1973, 81 (3): 637- 654
doi: 10.1086/260062 |
| 3 |
Carr P , Wu L . The finite moment log stable process and option pricing. J Finance, 2003, 58 (2): 753- 777
doi: 10.1111/1540-6261.00544 |
| 4 |
Cartea Á . Derivatives pricing with marked point processes using tick-by-tick data. Quant Finance, 2013, 13 (1): 111- 123
doi: 10.1080/14697688.2012.661447 |
| 5 | Cartea Á , Del-Castillo-Negrete D . Fractional diffusion models of option prices in markets with jumps. Physica A, 2006, 374 (2): 749- 763 |
| 6 |
Chen W , Xu X , Zhu S . Analytically pricing European-style options under the modified BlackScholes equation with a spatial-fractional derivative. Q Appl Math, 2014, 72 (3): 597- 611
doi: 10.1090/S0033-569X-2014-01373-2 |
| 7 | Fasshauer G , Zhang J . On choosing "optimal" shape parameters for RBF approximation. Numer Algorithms, 2007, 45 (1/4): 345- 368 |
| 8 |
Fu Z , Reutskiy S , Sun H , et al. A robust kernel-based solver for variable-order time fractional PDEs under 2D/3D irregular domains. Appl Math Lett, 2019, 94, 105- 111
doi: 10.1016/j.aml.2019.02.025 |
| 9 |
Gao G , Sun Z , Zhang H . A new fractional numerical differention formula to approximate the Caputo fractional derivative and its applications. J Comput Phys, 2014, 259, 33- 50
doi: 10.1016/j.jcp.2013.11.017 |
| 10 |
Gao Q , Wu Z , Zhang S . Adaptive moving knots meshless method for simulating time dependent partial differential equations. Eng Anal Bound Elem, 2018, 96, 115- 122
doi: 10.1016/j.enganabound.2018.08.010 |
| 11 |
Jumarie G . Derivation and solutions of some fractional Black-Scholes equations in coarse-grained space and time. Application to Merton's optimal portfolio. Comput Math With Appl, 2010, 59 (3): 1142- 1164
doi: 10.1016/j.camwa.2009.05.015 |
| 12 | Liang J , Wang J , Zhang W , et al. The solutions to a bi-fractional Black-Scholes-Merton differential equation. Int J Pure Appl Math, 2010, 58 (1): 99- 112 |
| 13 |
Ma L , Wu Z . Approximation to the k-th derivatives by multiquadric quasi-interpolation method. J Comput Appl Math, 2009, 231 (2): 925- 932
doi: 10.1016/j.cam.2009.05.017 |
| 14 |
Wu Z , Schaback R . Shape preserving properties and convergence of univariate multiquadric quasi-interpolation. Acta Math Appl Sin, 1994, 10 (4): 441- 446
doi: 10.1007/BF02016334 |
| 15 | Wyss W . The fractional Black-Scholes equation. Fract Calc Appl Anal, 2000, 3 (1): 51- 61 |
| 16 | Zhang H , Liu F , Turner L , et al. Numerical solution of the time fractional Black-Scholes model governing European options. Comput Math With Appl, 2016, 71 (9): 1772- 1783 |
| 17 | Zhang S , Yang H , Yang Y . A multiquadric quasi-interpolations method for CEV option pricing model. J Comput Appl Math, 2019, 347, 1- 11 |
| [1] | Xiling Li,Fei Gao,Wenqin Li. Stability Analysis of Fractional-Order Hepatitis B Virus Infection Model With Immune Delay [J]. Acta mathematica scientia,Series A, 2021, 41(2): 562-576. |
| [2] | Cheng Ouyang,Weigang Wang,Jiaqi Mo. The Fractional Generalized Disturbed Thermal Wave Equation [J]. Acta mathematica scientia,Series A, 2020, 40(2): 452-459. |
| [3] | Luogen Yao,Gang Yang,Xiangqun Yang. Mean Correcting Martingale Measure for Exponential Semimartingale Market Models [J]. Acta mathematica scientia,Series A, 2019, 39(4): 932-941. |
| [4] | Congcong Xu,Zuoliang Xu. Option Pricing Method and Parameter Calibration for Jump-Diffusion Model [J]. Acta mathematica scientia,Series A, 2019, 39(3): 649-663. |
| [5] | XIAO Shuang, JIAN Ming, CHEN Ai-Xiang, JIAN Bei. Fuzzy Pricing Formula for European Options with Jumps and Transaction Costs [J]. Acta mathematica scientia,Series A, 2015, 35(1): 118-130. |
| [6] | YANG Yin, CHEN Yan-Ping, HUANG Yun-Qing. CONVERGENCE ANALYSIS OF THE JACOBI SPECTRAL-COLLOCATION METHOD FOR FRACTIONAL INTEGRO-DIFFERENTIAL EQUATIONS [J]. Acta mathematica scientia,Series A, 2014, 34(3): 673-690. |
| [7] | SUN Yu-Dong, SHI Xi-Min, WU Min. Barrier Options Pricing when Parameters Dependent on Stock Price [J]. Acta mathematica scientia,Series A, 2013, 33(5): 912-925. |
| [8] | XIAO Wei-Wei, LIU Yong-Ping. Relative Widths of Function Classes of L2(T) Determined by Fractional Order Derivatives in Lq(T) [J]. Acta mathematica scientia,Series A, 2009, 29(4): 833-842. |
| [9] | Liu Shaoyue; Yang Xiangqun. Optimal Portfolio in a Fractional Black-Scholes Model with Arbitrary Hurst Parameter [J]. Acta mathematica scientia,Series A, 2008, 28(4): 742-746. |
| [10] |
Jian Xiongfei;Yi Fahuai.
The Obstacle Problem for the Pricing of the Fixed Rate Mortgages [J]. Acta mathematica scientia,Series A, 2007, 27(6): 987-995. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 162
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 86
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|