Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (2): 605-620.
Previous Articles Next Articles
A Modified HS-DY-Type Method with Nonmonotone Line Search for Image Restoration and Unconstrained Optimization Problems
Gonglin Yuan1( ),Yulun Wu1,*(
),Yulun Wu1,*( ),Hongtruong Pham2(
),Hongtruong Pham2( )
)
- 1 Center for Applied Mathematics of Guangxi & College of Mathematics and Information Science, Guangxi University, Nanning 530004
2 Thai Nguyen University of Economics and Business Administration, Thai Nguyen, Vietnam
-
Received:2021-03-03Online:2022-04-26Published:2022-04-18 -
Contact:Yulun Wu E-mail:glyuan@gxu.edu.cn;wuyulun@st.gxu.edu.cn;shanghaichina888@yahoo.com -
Supported by:the NSFC(11661009);the High Level Innovation Teams and Excellent Scholars Program in Guangxi Institutions of Higher Education([2019]52);the Guangxi Natural Science Key Fund(2017GXNSFDA198046);the Special Funds for Local Science and Technology Development Guided by the Central Government(ZY20198003);the Special Foundation for Guangxi Ba Gui Scholars
CLC Number:
- O221
Cite this article
Gonglin Yuan,Yulun Wu,Hongtruong Pham. A Modified HS-DY-Type Method with Nonmonotone Line Search for Image Restoration and Unconstrained Optimization Problems[J].Acta mathematica scientia,Series A, 2022, 42(2): 605-620.
share this article
"
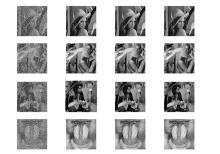
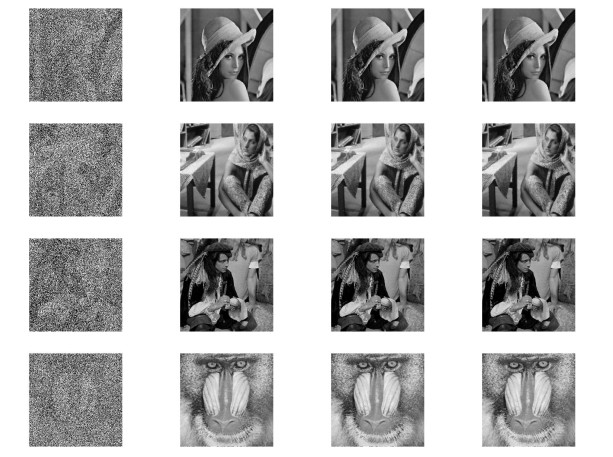
| 25%噪音浓度 | Lena | Barbara | Man | Baboom | 合计 |
| 方法1 | 6.016 | 31.188 | 7.781 | 7.281 | 52.266 |
| 方法4 | 6.563 | 33.094 | 8.641 | 7.75 | 56.048 |
| 方法5 | 6.359 | 33.578 | 8.859 | 7.515 | 56.311 |
| 50%噪音浓度 | Lena | Barbara | Man | Baboom | 合计 |
| 方法1 | 15.016 | 69.453 | 18.5 | 16.984 | 119.953 |
| 方法4 | 15.578 | 71.39 | 19.203 | 18.219 | 124.39 |
| 方法5 | 16.078 | 70.234 | 18.703 | 17.906 | 122.921 |
| 75% 噪音浓度 | Lena | Barbara | Man | Baboom | 合计 |
| 方法1 | 25.718 | 127.641 | 27.438 | 26.75 | 207.547 |
| 方法4 | 26.953 | 129.938 | 28.828 | 27.406 | 213.125 |
| 方法5 | 27.719 | 127.609 | 28.313 | 27.125 | 210.766 |
| 1 |
Dai Y H . New properties of a nonlinear conjugate gradient method. Numerische Mathematik, 2001, 89 (1): 83- 98
doi: 10.1007/PL00005464 |
| 2 |
Grippo L , Lucidi S . A globally convergent version of the Polak-Ribière conjugate gradient method. Mathematical Programming, 1997, 78 (3): 375- 391
doi: 10.1007/BF02614362 |
| 3 | Nocedal J , Wright S . Numerical Optimization. Berlin: Springer, 2006 |
| 4 | Shi Z . Restricted PR conjugate gradient method and its global convergence. Advances in Mathematics, 2002, 31: 47- 55 |
| 5 | Yuan G L , Wang X B , Sheng Z . Family weak conjugate gradient algorithms and their convergence analysis for nonconvex functions. Numerical Algorithms, 2002, 84 (3): 935- 956 |
| 6 |
Wei Z X , Yao S W , Liu L . The convergence properties of some new conjugate gradient methods. Applied Mathematics and Computation, 2006, 183 (2): 1341- 1350
doi: 10.1016/j.amc.2006.05.150 |
| 7 |
Zhou W . A short note on the global convergence of the unmodified PRP method. Optimization Letters, 2013, 7 (6): 1367- 1372
doi: 10.1007/s11590-012-0511-7 |
| 8 | Yuan G L , Lu J Y , Wang Z . The PRP conjugate gradient algorithm with a modified WWP line search and its application in the image restoration problems. Applied Numerical Mathematics, 2002, 152: 1- 11 |
| 9 |
Zhou W , Wang F . A PRP-based residual method for large-scale monotone nonlinear equations. Applied Mathematics and Computation, 2015, 261: 1- 7
doi: 10.1016/j.amc.2015.03.069 |
| 10 |
Yuan G L , Wei Z X , Yang Y N . The global convergence of the Polak-Ribière-Polyak conjugate gradient algorithm under inexact line search for nonconvex functions. Journal of Computational and Applied Mathematics, 2019, 362: 262- 275
doi: 10.1016/j.cam.2018.10.057 |
| 11 |
Wen F , Yang X . Skewness of return distribution and coefficient of risk premium. Journal of Systems Science and Complexity, 2009, 22 (3): 360- 371
doi: 10.1007/s11424-009-9170-x |
| 12 | Andrei N . An unconstrained optimization test functions collection. Advanced Modeling Optimization, 2008, 10 (1): 147- 161 |
| 13 |
Gilbert J , Nocedal J . Global convergence properties of conjugate gradient methods for optimization. SIAM Journal on Optimization, 1992, 2 (1): 21- 42
doi: 10.1137/0802003 |
| 14 |
Wei Z X , Li G Y , Qi L Q . Global convergence of the Polak-Ribiere-Polyak conjugate gradient method with an Armijo-type inexact line search for nonconvex unconstrained optimization problems. Mathematics of Computation, 2008, 77 (264): 2173- 2193
doi: 10.1090/S0025-5718-08-02031-0 |
| 15 |
Yu G , Guan L , Wei Z . Globally convergent Polak-Ribière-Polyak conjugate gradient methods under a modified Wolfe line search. Applied Mathematics and Computation, 2009, 215 (8): 3082- 3090
doi: 10.1016/j.amc.2009.09.063 |
| 16 |
Zhang L , Zhou W , Li D . Global convergence of a modified Fletcher-Reeves conjugate gradient method with Armijo-type line search. Numerische mathematik, 2006, 104 (4): 561- 572
doi: 10.1007/s00211-006-0028-z |
| 17 |
Li X , Ruan Q . A modified PRP conjugate gradient algorithm with trust region for optimization problems. Numerical Functional Analysis and Optimization, 2011, 32 (5): 496- 506
doi: 10.1080/01630563.2011.554948 |
| 18 |
Awwal A M , Kumam P , Wang L , et al. On the Barzilai-Borwein gradient methods with structured secant equation for nonlinear least squares problems. Optimization Methods and Software, 2020,
doi: 10.1080/10556788.2020.1855170 |
| 19 |
Grippo L , Lampariello F , Lucidi S . A nonmonotone line search technique for Newton's method. SIAM Journal on Numerical Analysis, 1986, 23 (4): 707- 716
doi: 10.1137/0723046 |
| 20 |
Grippo L , Lampariello F , Lucidi S . A truncated Newton method with nonmonotone line search for unconstrained optimization. Journal of Optimization Theory and Applications, 1989, 60 (3): 401- 419
doi: 10.1007/BF00940345 |
| 21 |
Toint P L . An assessment of nonmonotone linesearch techniques for unconstrained optimization. SIAM Journal on Scientific Computing, 1996, 17 (3): 725- 739
doi: 10.1137/S106482759427021X |
| 22 | Yahaya M M , Kumam P , Awwal A M , et al. A structured quasi-Newton algorithm with nonmonotone search strategy for structured NLS problems and its application in robotic motion control. Journal of Computational and Applied Mathematics, 2021, 395 (5): 113582 |
| 23 |
Zhang H , Hager W W . A nonmonotone line search technique and its application to unconstrained optimization. SIAM Journal on Optimization, 2004, 14 (4): 1043- 1056
doi: 10.1137/S1052623403428208 |
| 24 | Zhong W , Dong D F . Investigation on a class of nonmonotone cautious BFGS algorithms. Mathematica Numerica Sinica, 2011, 33 (4): 387- 396 |
| 25 |
Dai Y H . On the nonmonotone line search. Journal of Optimization Theory and Applications, 2002, 112 (2): 315- 330
doi: 10.1023/A:1013653923062 |
| 26 |
Amini K , Ahookhosh M , Nosratipour H . An inexact line search approach using modified nonmonotone strategy for unconstrained optimization. Numerical Algorithms, 2014, 66 (1): 49- 78
doi: 10.1007/s11075-013-9723-x |
| 27 |
Zhang L , Zhou W , Li D . Global convergence of the DY conjugate gradient method with Armijo line search for unconstrained optimization problems. Optimisation Methods and Software, 2007, 22 (3): 511- 517
doi: 10.1080/10556780600795748 |
| 28 |
Huang S , Wan Z , Chen X . A new nonmonotone line search technique for unconstrained optimization. Numerical Algorithms, 2015, 68 (4): 671- 689
doi: 10.1007/s11075-014-9866-4 |
| 29 | Hestenes M , Stiefel E . Methods of conjugate gradients for solving linear systems. Journal of Research of the National Bureau of Standards, 1952, 49 (1): 409- 436 |
| 30 |
Fletcher R , Reeves C M . Function minimization by conjugate gradients. The Computer Journal, 1964, 7 (2): 149- 154
doi: 10.1093/comjnl/7.2.149 |
| 31 | Polak E , Ribiere G . Note sur la convergence de méthodes de directions conjuguées. ESAIM: Mathematical Modelling and Numerical Analysis-Modélisation Mathématique et Analyse Numérique, 1969, 3 (R1): 35- 43 |
| 32 |
Polyak B T . The conjugate gradient method in extremal problems. USSR Computational Mathematics and Mathematical Physics, 1969, 9 (4): 94- 112
doi: 10.1016/0041-5553(69)90035-4 |
| 33 |
Liu Y , Storey C . Efficient generalized conjugate gradient algorithms, part 1: theory. Journal of Optimization Theory and Applications, 1991, 69 (1): 129- 137
doi: 10.1007/BF00940464 |
| 34 | Fletcher R . Practical Method of Optimization Vol I: Unconstrained Optimization. New York: John Wiley and Sons, 1987 |
| 35 |
Dai Y H , Yuan Y . A nonlinear conjugate gradient method with a strong global convergence property. SIAM Journal on Optimization, 1999, 10 (1): 177- 182
doi: 10.1137/S1052623497318992 |
| 36 | Zoutendijk G. Nonlinear Programming, Computational Methods//Abadie J. Integer and Nonlinear Programming. Amersterdam: North-Hollend, 1970: 37-86 |
| 37 |
Powell M J D . Convergence properties of algorithms for nonlinear optimization. Siam Review, 1986, 28 (4): 487- 500
doi: 10.1137/1028154 |
| 38 |
Hager W W , Zhang H . A new conjugate gradient method with guaranteed descent and an efficient line search. SIAM Journal on Optimization, 2005, 16 (1): 170- 192
doi: 10.1137/030601880 |
| 39 | Dai Y , Yuan Y . An efficient hybrid conjugate gradient method for unconstrained optimization. Annals of Operations Research, 2001, 103 (1): 33- 47 |
| 40 |
Liu J , Feng Y . A derivative-free iterative method for nonlinear monotone equations with convex constraints. Numerical Algorithms, 2019, 82 (1): 245- 262
doi: 10.1007/s11075-018-0603-2 |
| 41 |
Zhang L , Zhou W , Li D H . A descent modified Polak-Ribière-Polyak conjugate gradient method and its global convergence. IMA Journal of Numerical Analysis, 2006, 26 (4): 629- 640
doi: 10.1093/imanum/drl016 |
| 42 |
Abubakar A B , Kumam P , Ibrahim A H , et al. Derivative-free HS-DY-type method for solving nonlinear equations and image restoration. Heliyon, 2020, 6 (11): e05400
doi: 10.1016/j.heliyon.2020.e05400 |
| 43 |
Yuan G L , Wei Z X . Convergence analysis of a modified BFGS method on convex minimizations. Computational optimization and applications, 2010, 47 (2): 237- 255
doi: 10.1007/s10589-008-9219-0 |
| 44 | Yuan Y , Sun W . Theory and Methods of Optimization. Beijing: Science Press, 1999 |
| 45 |
Dolan E D , Moré J J . Benchmarking optimization software with performance profiles. Mathematical Programming, 2002, 91 (2): 201- 213
doi: 10.1007/s101070100263 |
| [1] | Xianzhen Jiang,Wei Liao,Jinbao Jian,Xiaodi Wu. An Improved PRP Type Spectral Conjugate Gradient Method with Restart Steps [J]. Acta mathematica scientia,Series A, 2022, 42(1): 216-227. |
| [2] | Zhibin Zhu,Yuanhang Geng. A Modified Three-Term WYL Conjugate Gradient Method [J]. Acta mathematica scientia,Series A, 2021, 41(6): 1871-1879. |
| [3] | Guodong Ma. Improved PRP and HS Conjugate Gradient Methods with the Strong Wolfe Line Search [J]. Acta mathematica scientia,Series A, 2021, 41(3): 837-847. |
| [4] | Yueyuan Liu,Kai Wang,Shujuan Qin,Jiaofen Li. An Effective Algorithm for Generalized Sylvester Equation Minimization Problem Under Columnwise Orthogonal Constraints [J]. Acta mathematica scientia,Series A, 2021, 41(2): 479-495. |
| [5] | Xiaoni Chi,Rong Zeng,Sanyang Liu,Zhibin Zhu. A Regularized Nonmonotone Inexact Smoothing Newton Algorithm for Weighted Symmetric Cone Complementarity Problems [J]. Acta mathematica scientia,Series A, 2021, 41(2): 507-522. |
| [6] | Liu Yang,Zuicha Deng. An Inverse Initial Value Problem for Degenerate Parabolic Equations [J]. Acta mathematica scientia,Series A, 2020, 40(4): 891-903. |
| [7] | Guodong Ma. A Strongly Convergent Generalized Gradient Projection Method for Minimax Optimization with General Constraints [J]. Acta mathematica scientia,Series A, 2020, 40(3): 641-649. |
| [8] | Xiangli Li,Juanjuan Shi,Xiaoliang Dong. A Class of Modified Non-Monotonic Spectral Conjugate Gradient Method and Applications to Non-Negative Matrix Factorization [J]. Acta mathematica scientia,Series A, 2018, 38(5): 954-962. |
| [9] | SONG Wei-Hong, ZHANG Kai-Yuan, NIE Yu-Feng. Double Iterative Algorithm for Different Constrained Solution of Discrete Coupled Algebraic Riccati Equation [J]. Acta mathematica scientia,Series A, 2014, 34(6): 1440-1449. |
| [10] | HU Chao-Ming, WAN Zhong, WANG Xu. A New Nonmonotone Spectral Conjugate Gradient Algorithm [J]. Acta mathematica scientia,Series A, 2013, 33(1): 78-88. |
| [11] | TANG Jing-Yong, HE Guo-Ping. A One-step Smoothing Newton Method for Second-order Cone Programming [J]. Acta mathematica scientia,Series A, 2012, 32(4): 768-778. |
| [12] | SUN Qing-Ying, DONG Jie-Hong, SANG Zhao-Yang. A New Trust Region Algorithm with Simple Quadratic Models and Line Search [J]. Acta mathematica scientia,Series A, 2010, 30(6): 1562-1574. |
| [13] | Xing Li-Li, LI Wei-Guo. A New Model for Staircase Reduction in Image Restoration Problems [J]. Acta mathematica scientia,Series A, 2009, 29(4): 882-890. |
| [14] | Zhang Li; Zhou Weijun. On the Global Convergence of the Hager-Zhang Conjugate Gradient Method with Armijo Line Search [J]. Acta mathematica scientia,Series A, 2008, 28(5): 840-845. |
| [15] |
Tong Xiaojiao ;He Wei.
Lagrangian Globalization Projection Methods for Nonlinear Constrained Equations [J]. Acta mathematica scientia,Series A, 2008, 28(1): 96-108. |
|
||