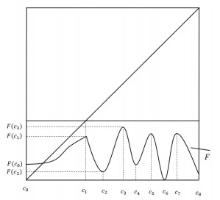
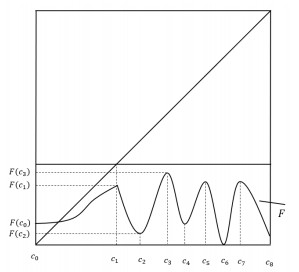
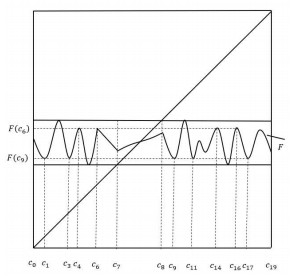
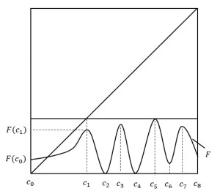
Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (2): 557-569.
Previous Articles Next Articles
Characteristic Endpoints Question for Piecewise Monotone Functions
- 1 School of Mathematical Science, Chongqing Normal University, Chongqing 401331
2 Faculty of Mathematics, Jiaxing University, Zhejiang Jiaxing 314001
-
Received:2021-02-01Online:2022-04-26Published:2022-04-18 -
Contact:Lin Li E-mail:mathll@163.com -
Supported by:the NSFC(12001537);the NSFC(11671061);the NSFC(12026207);the NSF of Zhejiang Province(LY18A010017);the PhD Start-up Fund of Chongqing Normal University(20XLB033)
CLC Number:
- O175
Cite this article
Xiao Tang,Lin Li. Characteristic Endpoints Question for Piecewise Monotone Functions[J].Acta mathematica scientia,Series A, 2022, 42(2): 557-569.
share this article
| 1 | Babbage C . An Essay towards the calculus of functions. Philos Trans R Soc Lond, 1815, 105: 389- 423 |
| 2 |
Baron K , Jarczyk W . Recent results on functional equations in a single variable, perspectives and open problems. Aequationes Math, 2001, 61: 1- 48
doi: 10.1007/s000100050159 |
| 3 |
Fort M . The embedding of homeomorphisms in flows. Proc Amer Math Soc, 1955, 6: 960- 967
doi: 10.1090/S0002-9939-1955-0080911-2 |
| 4 | Irwin M C . Smooth Dynamical Systems. New York: Academic Press, 1980 |
| 5 |
Kuczma M . On the functional equation φn(x)=g(x). Ann Polon Math, 1961, 11: 161- 175
doi: 10.4064/ap-11-2-161-175 |
| 6 | Kuczma M . Functional Equations in a Single Variable. Warszawa: Polish Scientific Publishers, 1968 |
| 7 | Kuczma M , Choczewski B , Ger R . Iterative Functional Equations. Cambridge: Cambridge University Press, 1990 |
| 8 |
Li L , Chen J . Iterative roots of piecewise monotonic functions with finite nonmonotonicity height. J Math Anal Appl, 2014, 411: 395- 404
doi: 10.1016/j.jmaa.2013.09.051 |
| 9 |
Li L , Yang D L , Zhang W N . A note on iterative roots of PM functions. J Math Anal Appl, 2008, 341: 1482- 1486
doi: 10.1016/j.jmaa.2007.11.006 |
| 10 |
Li L , Zhang W N . The question on characteristic endpoints for iterative roots of PM functions. J Math Anal Appl, 2018, 458: 265- 280
doi: 10.1016/j.jmaa.2017.09.009 |
| 11 |
Liu L , Jarczyk W , Li L , Zhang W N . Iterative roots of piecewise monotonic functions of nonmonotonicity height not less than 2. Nonlinear Anal, 2012, 75: 286- 303
doi: 10.1016/j.na.2011.08.033 |
| 12 | Palis J , Melo W . Geometric Theory of Dynamical Systems: An Introduction. New York: Springer, 1982 |
| 13 |
Solarz P . General theorem for the existence of iterative roots of homeomorphisms with periodic points. J Math Anal Appl, 2012, 394: 391- 399
doi: 10.1016/j.jmaa.2012.03.048 |
| 14 | Thompson J M T , Stewart H B . Nonlinear Dynamics and Chaos. Chichester: John Wiley & Sons, 1986 |
| 15 |
Zdun M , Solarz P . Recent results on iteration theory: iteration groups and semigroups in the real case. Aequationes Math, 2014, 87: 201- 245
doi: 10.1007/s00010-013-0186-x |
| 16 | 张景中, 杨路. 论逐段单调连续函数的迭代根. 数学学报, 1983, 26 (4): 398- 412 |
| 17 |
Zhang W N . PM functions, their characteristic intervals and iterative roots. Ann Polon Math, 1997, 65: 119- 128
doi: 10.4064/ap-65-2-119-128 |
| [1] | Shijie Shi,Zhengrong Liu,Hui Zhao. Boundedness and Stabilization of a Chemotaxis Model Describing Tumor Invasion with Signal-Dependent Motility [J]. Acta mathematica scientia,Series A, 2022, 42(2): 502-519. |
| [2] | Hongmei Cheng,Rong Yuan. Forced Waves of a Delayed Reaction-Diffusion Equation with Nonlocal Diffusion Under Shifting Environment [J]. Acta mathematica scientia,Series A, 2022, 42(2): 491-501. |
| [3] | Weiqiang Zhang,Peihao Zhao. Existence, Multiplicity and Concentration of Positive Solutions for a Fractional Choquard Equation [J]. Acta mathematica scientia,Series A, 2022, 42(2): 470-490. |
| [4] | Jun Lan. Existence and Multiplicity of Anti-Periodic Solutions for a Class of Second Order Duffing Equation [J]. Acta mathematica scientia,Series A, 2022, 42(2): 463-469. |
| [5] | Shaowen Yao,Wenjie Li,Zhibo Cheng. Nondegeneracy and Uniqueness of Periodic Solution for Third-Order Nonlinear Differential Equations [J]. Acta mathematica scientia,Series A, 2022, 42(2): 454-462. |
| [6] | Mengxue Du,Fanghui Li,Zhengping Wang. Multiplicity of Normalized Solutions for Nonlinear Schrödinger-Poisson Equation with Hardy Potential [J]. Acta mathematica scientia,Series A, 2022, 42(2): 442-453. |
| [7] | Kexin Luo,Shaoyong Lai. Global Weak Solutions to a High-Order Camass-Holm Type Equation [J]. Acta mathematica scientia,Series A, 2022, 42(2): 427-441. |
| [8] | Die Hu,Qi Gao. Multiple Solutions to Logarithmic Kirchhoff Equations [J]. Acta mathematica scientia,Series A, 2022, 42(2): 401-417. |
| [9] | Tong Tang,Cong Niu. Global Existence of Weak Solutions to the Quantum Navier-Stokes Equations [J]. Acta mathematica scientia,Series A, 2022, 42(2): 387-400. |
| [10] | Lei Duan,Tianlan Chen. Existence of Convex Solutions for a Discrete Mixed Boundary Value Problem with the Mean Curvature Operator [J]. Acta mathematica scientia,Series A, 2022, 42(2): 379-386. |
| [11] | Xueli Jiang,Xuan Deng,Qiuhao Wen,Yanqin Xiong. Limit Circle Bifurcations of Polynomial Differential System with Two Parallel Switch Straight Lines [J]. Acta mathematica scientia,Series A, 2022, 42(2): 353-364. |
| [12] | Zhang Daoxiang, Li Ben, Chen Dandan, Lin Yating, Wang Xinmei. Hopf Bifurcation for a Fractional Differential-Algebraic Predator-Prey System with Time Delay and Economic Profit [J]. Acta mathematica scientia,Series A, 2022, 42(2): 570-582. |
| [13] | Changyou Wang,Nan Li,Tao Jiang,Qiang Yang. On a Nonlinear Non-Autonomous Ratio-Dependent Food Chain Model with Delays and Feedback Controls [J]. Acta mathematica scientia,Series A, 2022, 42(1): 245-268. |
| [14] | Min Zhu,Mengli Liu. A Dengue Fever Model Incorporating Heterogeneous Cross-Diffusion [J]. Acta mathematica scientia,Series A, 2022, 42(1): 201-215. |
| [15] | Tong Zhao,Hailong Yuan,Gaihui Guo. Positive Solutions of a Predator-Prey Model with Modified Leslie-Gower Type [J]. Acta mathematica scientia,Series A, 2022, 42(1): 176-186. |
|
||