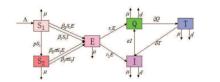
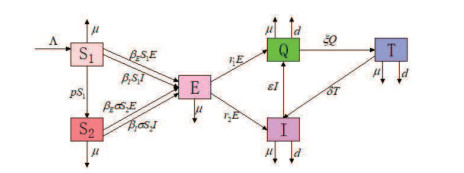
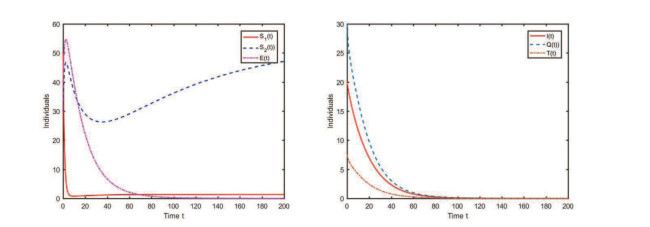
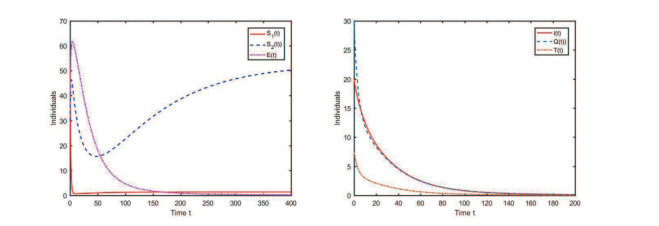
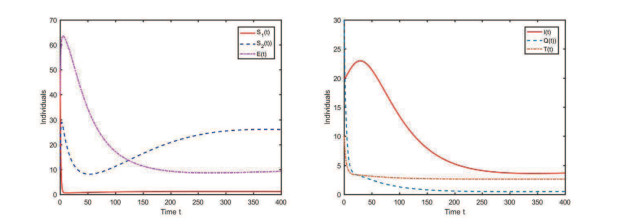
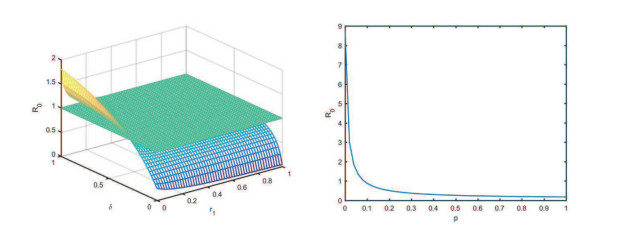
Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (4): 1235-1248.
Global Stability of an Epidemic Model with Quarantine and Incomplete Treatment
- Ningxia Institute of Science and Technology, Ningxia Shizuishan 753000
-
Received:2020-06-11Online:2021-08-26Published:2021-08-09 -
Contact:Defen Wang E-mail:261337439@qq.com;wangdefen_2008@163.com -
Supported by:the NSF of Ningxia Hui Autonomous Region(2021AAC03256)
CLC Number:
- O175.1
Cite this article
Lixiang Feng,Defen Wang. Global Stability of an Epidemic Model with Quarantine and Incomplete Treatment[J].Acta mathematica scientia,Series A, 2021, 41(4): 1235-1248.
share this article
| 1 |
Zhou P , Yang X L , Wang X G , et al. A pneumonia outbreak associated with a new coronavirus of probable bat origin. Nature, 2020, 579, 270- 273
doi: 10.1038/s41586-020-2012-7 |
| 2 |
Li Q , Guan X , Wu P , et al. Early transmission dynamics in Wuhan, China, of Noval Coronavirus-infected Pneumonia. N Engl J Med, 2020, 382, 1199- 1207
doi: 10.1056/NEJMoa2001316 |
| 3 |
Gao Q W , Zhuang J . Stability analysis and control strategies for worm attack in mobile networks via a VEIQS propagation model. Appl Math Comput, 2020,
doi: 10.1016/j.amc.2019.124584 |
| 4 | 邢伟, 高晋芳, 颜七笙, 等. 一类受媒体报道影响的SEIS传染病模型的定性分析. 西北大学学报(自然科学版), 2018, 48 (5): 639- 643 |
| Xing W , Gao J F , Yan Q S , et al. An epidemic model with saturated media/psychological impact. Journal of Northwest University (Natural Science Edition), 2018, 48 (5): 639- 643 | |
| 5 | 严阅, 陈瑜, 刘可伋, 等. 基于一类时滞动力学系统对新型冠状病毒肺炎疫情的建模和预测. 中国科学: 数学, 2020, 50 (3): 385- 392 |
| Yan Y , Chen Y , Liu K , et al. Modeling and prediction for the trend of outbreak of NCP based on a time-delay dynamic system (in Chinese). Sci Sin Math, 2020, 50 (3): 385- 392 | |
| 6 | 王霞, 唐三一, 陈勇, 等. 新型冠状病毒肺炎疫情下武汉及周边地区何时复工? 数据驱动的网络模型分析. 中国科学: 数学, 2020, 50 (7): 969- 978 |
| Wand X , Tang S Y , Chen Y , et al. When will be the resumption of work in wuhan and its surrounding areas during COVID-19 epidemic? A data-driven network modeling analysis (in Chinese). Sci Sin Math, 2020, 50 (7): 969- 978 | |
| 7 | Xing Y , Zhang L , Wang X . Modeling and stability of epidemic model with free-living pathogens growing in the environment. J Appl Anal Comput, 2020, 10 (1): 55- 70 |
| 8 |
Liu S , Zhang L , Xing Y . Dynamics of a stochastic heroin epidemic model. J Comput Appl Math, 2019, 351, 260- 269
doi: 10.1016/j.cam.2018.11.005 |
| 9 |
邓栋, 李燕. 一类带治疗项的非局部扩散SIR传染病模型的行波解. 数学物理学报, 2020, 40A (1): 72- 102
doi: 10.3969/j.issn.1003-3998.2020.01.008 |
|
Deng D , Li Y . Traveling waves in a nonlocal dispersal SIR epidemic model with treatment. Acta Math Sci, 2020, 40A (1): 72- 102
doi: 10.3969/j.issn.1003-3998.2020.01.008 |
|
| 10 |
曹忠威, 文香丹, 冯徽, 等. 一类具有随机扰动的非自治SIRI流行病模型的动力学行为. 数学物理学报, 2020, 40A (1): 221- 233
doi: 10.3969/j.issn.1003-3998.2020.01.017 |
|
Cao Z W , Wen X D , Feng W , et al. Dynamics of a nonautonomous SIRI epidemic model with random perturbations. Acta Math Sci, 2020, 40A (1): 221- 233
doi: 10.3969/j.issn.1003-3998.2020.01.017 |
|
| 11 |
Huo H F , Feng L X . Global stability for an HIV/AIDS epidemic model with different latent stages and treatment. Applied Mathematical Modelling, 2013, 37 (3): 1480- 1489
doi: 10.1016/j.apm.2012.04.013 |
| 12 |
Du Z W , Xu X K , Wu Y , et al. The serial interval of COVID-19 among publicly reported confirmed cases. medRxiv, 2020,
doi: 10.1101/2020.02.19.20025452 |
| 13 |
霍海峰, 邹明轩. 一类具有接种和隔离治疗的结核病模型的稳定性. 兰州理工大学学报, 2016, 42 (3): 150- 154
doi: 10.3969/j.issn.1673-5196.2016.03.030 |
|
Huo H F , Zou M X . Stability of a tuberculosis model with vaccination and isolation treatment. Journal of Lanzhou University of Technology, 2016, 42 (3): 150- 154
doi: 10.3969/j.issn.1673-5196.2016.03.030 |
|
| 14 | 张菊平, 李云, 靳祯, 等. 武汉市COVID-19疫情与易感人群软隔离强度关系分析. 应用数学学报, 2020, 43 (2): 162- 173 |
| Zhang J P , Li Y , Jin Z , et al. Analysis of the relationship between transmission of COVID-19 in Wuhan and soft quarantine intensity in susceptible population. Acta Math Appl, 2020, 43 (2): 162- 173 | |
| 15 | 李倩, 肖燕妮, 唐三一, 等. COVID-19疫情时滞模型构建与确诊病例驱动的追踪隔离措施分析. 应用数学学报, 2020, 43 (2): 238- 250 |
| Li Q , Xiao Y N , Tang S Y , et al. Modelling COVID-19 epidemic with time delay and analyzing the strategy of confirmed cases-driven contact tracing followed by quarantine. Acta Math Appl, 2020, 43 (2): 238- 250 | |
| 16 | Yang Y L , Li J Q , Ma Z E , Liu L J . Global stability of two models with incomplete for tuberculosis. Chaos, Solitons & Fractals, 2010, 43, 79- 85 |
| 17 |
Huo H F , Feng L X . Global stability of an epidemic model with incomplete treatment and vaccination. Discrete Dynamics in Nature and Society, 2012,
doi: 10.1155/2012/530267 |
| 18 | Yang X , Chen L . Permanence and positive periodic solution for the single-species nonautonomous delay diffusive model. Comput Math Appl, 1996, 32 (4): 109- 116 |
| 19 |
van den Driessche P , Watmough J . Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math Biosci, 2002, 180, 29- 48
doi: 10.1016/S0025-5564(02)00108-6 |
| 20 | LaSalle J P. The Stability of Dynamical Systems, Regional Conference Series in Applied Mathematics. Philadelphia: SIAM, 1976 |
| [1] | Zhonghua Zhang,Qian Zhang. Qualitative Analysis of a Stochastic SIVS Epidemic Model with Nonlinear Perturbations Under Regime Switching [J]. Acta mathematica scientia,Series A, 2021, 41(4): 1218-1234. |
| [2] | Yonghui Zhou. Global Stability of the Nonmonotone Critical Traveling Waves for Reaction Diffusion Equations [J]. Acta mathematica scientia,Series A, 2020, 40(4): 983-992. |
| [3] | Xinxin Cheng,Yaqing Rao,Gang Huang. COVID-19 Transmission Model in an Enclosed Space: A Case Study of Japan Diamond Princess Cruises [J]. Acta mathematica scientia,Series A, 2020, 40(2): 540-544. |
| [4] | Zhongwei Cao,Xiangdan Wen,Wei Feng,Li Zu. Dynamics of a Nonautonomous SIRI Epidemic Model with Random Perturbations [J]. Acta mathematica scientia,Series A, 2020, 40(1): 221-233. |
| [5] | Xinzhe Zhang,Guofeng He,Gang Huang. Dynamical Properties of a Delayed Epidemic Model with Vaccination and Saturation Incidence [J]. Acta mathematica scientia,Series A, 2019, 39(5): 1247-1259. |
| [6] | Xiaojie Jing, Aimin Zhao, Guirong Liu. Global Stability of a Measles Epidemic Model with Partial Immunity and Environmental Transmission [J]. Acta mathematica scientia,Series A, 2019, 39(4): 909-917. |
| [7] | Zhang Liping, Zhao Yu, Yuan Sanling. Threshold Dynamical Behaviors of a Stochastic SIS Epidemic Model with Nonlinear Incidence Rate [J]. Acta mathematica scientia,Series A, 2018, 38(1): 197-208. |
| [8] | Meng Xiaoying. Analysis of a Stochastic Delayed Epidemic Model with a Non-Monotonic Incidence Rate [J]. Acta mathematica scientia,Series A, 2017, 37(6): 1162-1175. |
| [9] | Wei Fengying, Lin Qingteng. Extinction and Distribution for an SIQS Epidemic Model with Quarantined-Adjusted Incidence [J]. Acta mathematica scientia,Series A, 2017, 37(6): 1148-1161. |
| [10] | Wei Aiju, Zhang Xinjian, Wang Junyi, Li Kezan. Dynamics Analysis of an Ebola Epidemic Model [J]. Acta mathematica scientia,Series A, 2017, 37(3): 577-592. |
| [11] | Xu Yanli. Exponential Convergence of An Epidemic Model with Delays and Oscillating Recovery Rates [J]. Acta mathematica scientia,Series A, 2015, 35(5): 987-994. |
| [12] | LING Lin, LIU Su-Yu, JIANG Gui-Rong. Bifurcation Analysis of a SIRS Epidemic Model with Saturating Contact Rate and Vertical Transmission [J]. Acta mathematica scientia,Series A, 2014, 34(6): 1415-1425. |
| [13] | YE Hui, CAI Dong-Han. Global Attractivity in a Periodic Nicholson's Blowflies Model [J]. Acta mathematica scientia,Series A, 2013, 33(6): 1013-1021. |
| [14] | LI Yu-Huan, ZHOU Jun, MU Chun-Lai. Stability Analysis for a Predator-Prey System with Nonlocal Delayed Reaction-diffusion Equations [J]. Acta mathematica scientia,Series A, 2012, 32(3): 475-488. |
| [15] | CUI Cheng, WANG Xiao, GAO Fei, LIU An-Ping. Global Stability of Periodic Solution and Almost Periodic Solution of Price Cooperating Model with Time Lag [J]. Acta mathematica scientia,Series A, 2011, 31(6): 1718-1729. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 227
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 103
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||||||
|