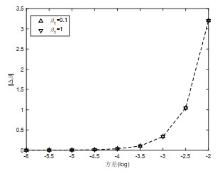
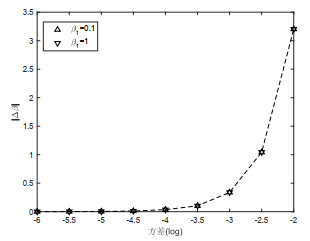
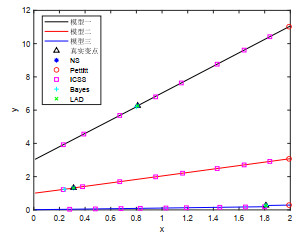
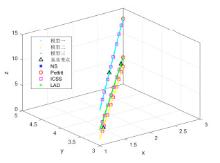
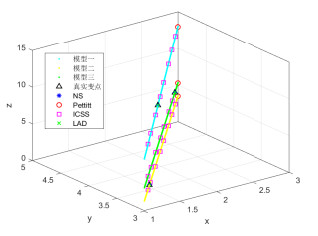
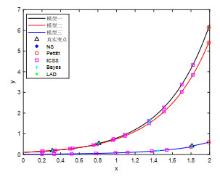
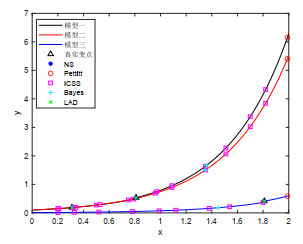
Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (4): 1124-1134.
Previous Articles Next Articles
Research on Change-Point Detection for Parameters in Regression Model
Jinhui Zheng1( ),Jinghu Yu1,*(
),Jinghu Yu1,*( ),Yiming Ding1(
),Yiming Ding1( ),Zeyu Bao2(
),Zeyu Bao2( )
)
- 1 School of Science, Wuhan University of Technology, Wuhan 430070
2 Junior Class College, University of Science and Technology of China, Hefei 230026
-
Received:2020-11-02Online:2021-08-26Published:2021-08-09 -
Contact:Jinghu Yu E-mail:zhengjh@whut.edu.cn;yujh67@126.com;dingym@whut.edu.cn;windy888@mail.ustc.edu.cn
CLC Number:
- O212
Cite this article
Jinhui Zheng,Jinghu Yu,Yiming Ding,Zeyu Bao. Research on Change-Point Detection for Parameters in Regression Model[J].Acta mathematica scientia,Series A, 2021, 41(4): 1124-1134.
share this article
| 1 |
Page E S . Continuous inspection schemes. Biometrika, 1954, 41 (1/2): 100- 115
doi: 10.2307/2333009 |
| 2 | Inclán C , Tiao G C . Use of cumulative sums of squares for retrospective detection of changes of variance. Journal of the American Statistical Association, 1994, 89 (427): 913- 923 |
| 3 | Goossens C , Berger A . Annual and seasonal climatic variations over the northern hemisphere and Europe during the last century. Annales Geophysicae, 1986, 4 (4): 385- 400 |
| 4 | Pettitt A N . A nonparametric approach to the change-point problem. Journal of the Royal Statistical Society: Series C (Applied Statistics), 1979, 28 (2): 126- 135 |
| 5 |
谭常春, 陈思, 缪柏其. 跳-斜度变点估计的强收敛速度. 中国科学技术大学学报, 2011, 41 (9): 773- 777
doi: 10.3969/j.issn.0253-2778.2011.09.004 |
|
Tan C C , Chen S , Miao B Q . The strong convergence rate of jump-slope change point estimation. Journal of University of Science and Technology of China, 2011, 41 (9): 773- 777
doi: 10.3969/j.issn.0253-2778.2011.09.004 |
|
| 6 | 谭智平, 缪柏其. 关于分布变点问题的非参数统计推断. 中国科学技术大学学报, 2000, 3, 21- 28 |
| Tan Z P , Miao B Q . Nonparametric statistical inference on the distribution change point. Journal of University of Science and Technology of China, 2000, 3, 21- 28 | |
| 7 | Oh H , Lee S . On score vector-and residual-based CUSUM tests in ARMA-GARCH models. Statistical Methods & Applications, 2018, 27 (3): 385- 406 |
| 8 |
叶五一, 缪柏其, 谭常春. 基于分位点回归模型变点检测的金融传染分析. 数量经济技术经济研究, 2007, 24 (10): 151- 160
doi: 10.3969/j.issn.1000-3894.2007.10.015 |
|
Ye W Y , Miao B Q , Tan C C . Analysis of financial contagion based on change point detection of quantile regression model. Quantitative and Technical Economic Research, 2007, 24 (10): 151- 160
doi: 10.3969/j.issn.1000-3894.2007.10.015 |
|
| 9 |
叶五一, 缪柏其. 基于Copula变点检测的美国次级债金融危机传染分析. 中国管理科学, 2009, 17 (3): 1- 7
doi: 10.3321/j.issn:1003-207X.2009.03.001 |
|
Ye W Y , Miao B Q . Analysis of the contagion of U.S. subprime debt financial crisis based on copula change coint detection. Chinese Management Science, 2009, 17 (3): 1- 7
doi: 10.3321/j.issn:1003-207X.2009.03.001 |
|
| 10 | Lattanzi C , Leonelli M . A change point approach for the identification of financial extreme regimes. Statistics, 2019, 19 (1): 1- 34 |
| 11 |
Perreault L , Bernier J , Bobée B , et al. Bayesian change-point analysis in hydrometeorological time series. Part 1:The normal model revisited. Journal of Hydrology, 2000, 235 (3-4): 221- 241
doi: 10.1016/S0022-1694(00)00270-5 |
| 12 |
Chen S , Li Y , Kim J , et al. Bayesian change point analysis for extreme daily precipitation. International Journal of Climatology, 2017, 37 (7): 3123- 3137
doi: 10.1002/joc.4904 |
| 13 |
Hu J . Cancer outlier detection based on likelihood ratio test. Bioinformatics, 2008, 24 (19): 2193- 2199
doi: 10.1093/bioinformatics/btn372 |
| 14 |
Wu D , Faria A V , Younes L , et al. Mapping the order and pattern of brain structural MRI changes using change-point analysis in premanifest Huntington's disease. Human Brain Mapping, 2017, 38 (10): 5035- 5050
doi: 10.1002/hbm.23713 |
| 15 |
Quandt , Richard E . The estimation of the parameters of a linear regression system obeying two separate regimes. Journal of the American Statistical Association, 1958, 53 (284): 873- 880
doi: 10.1080/01621459.1958.10501484 |
| 16 |
Quandt , Richard E . Tests of the hypothesis that a linear regression system obeys two separate regimes. Journal of the American Statistical Association, 1960, 55 (290): 324- 330
doi: 10.1080/01621459.1960.10482067 |
| 17 | Liu Zhihua , Qian Lianfen . Changepoint estimation in a segmented linear regression via empirical likelihood. Communications in Statistics-Simulation and Computation, 2010, 39 (1): 85- 100 |
| 18 |
Lee S , Seo M H , Shin Y . Testing for threshold effects in regression models. Journal of the American Statistical Association, 2011, 106 (493): 220- 231
doi: 10.1198/jasa.2011.tm09800 |
| 19 |
Bai J . Estimation of a change point in multiple regression models. Review of Economics and Statistics, 1997, 79 (4): 551- 563
doi: 10.1162/003465397557132 |
| 20 | 蒋家坤, 林华珍, 蒋靓, 等. 门槛回归模型中门槛值和回归参数的估计. 中国科学: 数学, 2016, 46 (4): 41- 54 |
| Jiang J K , Lin H Z , Jiang L , et al. Estimation of threshold and regression parameters in threshold regression model. Science in China: Mathematics, 2016, 46 (4): 41- 54 | |
| 21 | Tang Y C , Wang P P , Chen H . Bayesian analysis for change-point linear regression models. Chinese Journal of Applied Probability and Statistics, 2015, 31 (1): 89- 102 |
| 22 | Ciuperca G . The M-estimation in a multi-phase random nonlinear model. Stats & Probability Letters, 2009, 79 (5): 573- 580 |
| 23 | Ciuperca G . Estimating nonlinear regression with and without change-points by the LAD method. Annals of the Institute of Statal Mathematics, 2011, 63 (4): 717- 743 |
| 24 | Boldea O , Hall A R . Estimation and inference in unstable nonlinear least squares models. Journal of Econometrics, 2013, 172 (1): 158- 167 |
| 25 | 谭秋衡. 时间序列的非平稳性度量及其应用[D]. 北京: 中国科学院研究生院, 2013 |
| Tan Q H. Non-Stationary Measurement of Time Series and Its Application[D]. Beijing: Graduate School of Chinese Academy of Sciences, 2013 | |
| 26 | 谭秋衡, 丁义明. 基于非平稳性度量的彩票数据实证分析. 数学物理学报, 2014, 34 (1): 207- 216 |
| Tan Q H , Ding Y M . Empirical analysis of lottery data based on non-stationarity measure. Acta Math Sci, 2014, 34 (1): 207- 216 | |
| 27 | 谭秋衡, 吴量, 李波. 基于EMD及非平稳性度量的趋势噪声分解方法. 数学物理学报, 2016, 36 (4): 783- 794 |
| Tan Q H , Wu L , Li B . Decomposition of noise and trend based on EMD and non-stationarity measure. Acta Math Sci, 2016, 36 (4): 783- 794 |
| [1] | WANG Cheng-Yong. A Semiparametric Smooth Transition Regression Model and Its Series Estimator [J]. Acta mathematica scientia,Series A, 2012, 32(4): 797-807. |
| [2] | MA Tie-Feng, WANG Song-Gui. The Adjusted of Parameter Estimation in Seemingly Unrelated Regression [J]. Acta mathematica scientia,Series A, 2009, 29(5): 1256-1264. |
| [3] | FENG San-Ying, XUE Liu-Gen, FAN Cheng-Hua. Empirical Likelihood Confidence Regions of the Parameters in Nonlinear Semiparametric Regression Models [J]. Acta mathematica scientia,Series A, 2009, 29(5): 1338-1349. |
| [4] | Li Yuan;Yang Yidang. Testing Heteroscedasticity by Wavelets in a Nonparametric Regression Model with Random Design [J]. Acta mathematica scientia,Series A, 2007, 27(4): 727-740. |
| [5] |
Zhang Shangli;Qin Hong.
Influence Analysis of Covariance Matrix Disturbance in Linear Model with Respect to a Restricted Condition [J]. Acta mathematica scientia,Series A, 2006, 26(4): 621-628. |
| [6] |
Zhu Zhongyi ;Ran Hao.
Testing for Heteroscedasticity in Semiparametric |
| [7] | BO Guang-Ming, HU Shu-He, FANG Li-Bao, CHENG Zheng-Dong. Mean Consistency for a Semiparametric Regression Model [J]. Acta mathematica scientia,Series A, 2003, 23(5): 598-606. |
| [8] | Hu Shuhe. Estimate for a Semiparametric Regression Model [J]. Acta mathematica scientia,Series A, 1999, 19(5): 541-549. |
| [9] | Chen Minghua. Strong Consistency of Estimators in a Semi-Parametric Model with Completed and Censored Data [J]. Acta mathematica scientia,Series A, 1999, 19(5): 501-506. |
| [10] | Chen Minghua. The bootstrap approximation of paremetric estimator in a semii parametric model under fixed desiqn [J]. Acta mathematica scientia,Series A, 1999, 19(2): 121-129. |
| [11] | Lin Lu. Quasi Likelihood Estimation and Constrained Quasi Likelihood Estimation in Nonlinear Regression Model [J]. Acta mathematica scientia,Series A, 1998, 18(S1): 133-138. |
| [12] | Shi Lei, Ren Shiquan. ASSESSMENT OF LOCAL INFLUENCE IN MULTIVARIATE REGRESSION MODEL [J]. Acta mathematica scientia,Series A, 1997, 17(S1): 184-194. |
| Viewed | ||||||||||||||||||||||||||||||||||||||||||||||
|
Full text 152
|
|
|||||||||||||||||||||||||||||||||||||||||||||
|
Abstract 120
|
|
|||||||||||||||||||||||||||||||||||||||||||||
Cited |
|
|||||||||||||||||||||||||||||||||||||||||||||
| Shared | ||||||||||||||||||||||||||||||||||||||||||||||
| Discussed | ||||||||||||||||||||||||||||||||||||||||||||||
|
||