考虑部分免疫和环境传播的麻疹传染病模型的全局稳定性

Abstract

Abstract:

In this paper, a measles epidemic model with partial immunity and environmental transmission is considered, and the basic reproduction number R₀ is obtained. By constructing Lyapunov functions, we prove the global asymptotic stability of the infection-free equilibrium and the endemic equilibrium. When R₀ < 1, the infection-free equilibrium is globally asymptotically stable, which implies that measles dies out eventually; when R₀ > 1, the model has a unique endemic equilibrium, which is globally asymptotically stable, that is the transmission of measles keeps a steady state. Finally, the simulations are carried to verify the rationality of the results. This work has practical significance for guiding us to prevent and control the measles spread.

Key words: Partial immunity, Environmental transmission, The basic reproduction number, Lyapunov function, Global stability

CLC Number:

O175.1

Xiaojie Jing, Aimin Zhao, Guirong Liu. Global Stability of a Measles Epidemic Model with Partial Immunity and Environmental Transmission[J].Acta mathematica scientia,Series A, 2019, 39(4): 909-917.

Trendmd

Figures/Tables 4

References 13

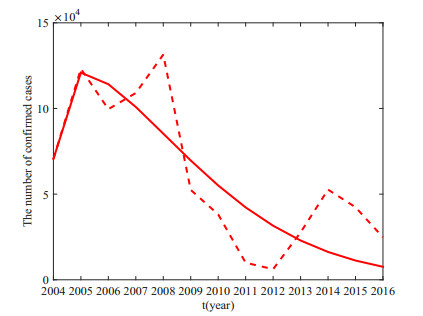
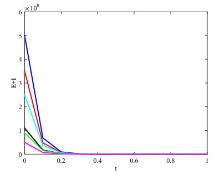
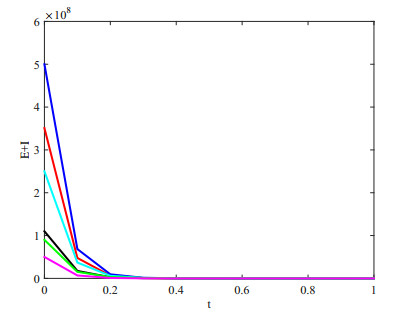
1	Liu X N , Takeuchi Y , Iwami S . SV IR epidemic models with vaccination strategies. J Theor Biol, 2008, 253 (1): 1- 11 doi: 10.1016/j.jtbi.2007.10.014
2	Mossong J , Muller C P . Modelling measles re-emergence as a result of waning of immunity in vaccinated populations. Vaccine, 2003, 21 (31): 4597- 4603 doi: 10.1016/S0264-410X(03)00449-3
3	Ejima K , Omori R . Real-time investigation of measles of vaccine efficacy. Int J Biol Sci, 2012, 8 (5): 620- 629 doi: 10.7150/ijbs.4329
4	Bolarin G . On the dynamical analysis of a new model for measles infection. IJMTT, 2014, 7 (2): 144- 155 doi: 10.14445/22315373/IJMTT-V7P519
5	姜翠翠, 宋丽娟, 王开发. 考虑部分免疫和潜伏期的麻疹传染病模型的稳定性分析. 生物数学学报, 2017, 32 (1): 57- 64
	Jiang C C , Song L J , Wang K F . Stability analysis of a measles epidemic model with partial immunity and latency. J Biomath, 2017, 32 (1): 57- 64
6	Garba S M , Safi M A , Usaini S . Mathematical model for assessing the impact of vaccination and treatment on measles transmission dynamics. Math Meth Appl Sci, 2017, 40 (18): 6371- 6388 doi: 10.1002/mma.4462
7	Trottier H , Philippe P . Deterministic modelling of infectious diseases:measles cycles and the role of births and vaccination. The Internet J of Infect Diseases, 2002, 2 (2): 1- 8
8	Trottier H , Philippe P . Deterministic modelling of infectious diseases:applications to measles and other similar infections. The Internet J of Infect Diseases, 2001, 2 (1): 1- 10
9	Huang J C , Ruan S G , Wu X , Zhou X L . Seasonal transmission dynamics of measles in China. Theor Biosci, 2018, 137 (2): 185- 195 doi: 10.1007/s12064-018-0271-8
10	Van den Driessche P , Watmough J . Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math Biosci, 2002, 180 (1-2): 29- 48 doi: 10.1016/S0025-5564(02)00108-6
11	Lasalle J P . The Stability of Dynamical Systems. Philadelphia: SIMA, 1976
12	马知恩, 周义仓, 李承治. 常微分方程定性与稳定性方法. 北京: 科学出版社, 2015
	Ma Z E , Zhou Y C , Li C Z . Qualitative and Stability of Ordinary Differential Equation. Beijing: Science Press, 2015
13	马知恩, 周义仓, 王稳地, 靳祯. 传染病动力学的数学建模与研究. 北京: 科学出版社, 2004
	Ma Z E , Zhou Y C , Wang W D , Jin Z . The Mathematical Modeling and Research of Infectious Diseases. Beijing: Science Press, 2004

参数	取值	意义	来源
$\lambda$	$1.728\times 10^{7}$	新生儿的出生率	国家统计局(2017)
$\mu$	0.0131	自然死亡率	国家统计局(2017)
$\eta$	0.8216	新生儿接种疫苗的比例	文献[9]
$\beta$	$9.6696\times 10^{-9}$	人与人的感染率	估计
$\alpha$	$1.1352\times 10^{-9}$	环境对人的感染率	估计
$\varepsilon$	0.15	无效的接种率	中国疾病预防控制中心(2017)
$\delta$	0.95	接种疫苗后获得免疫并成为恢复者的转移率	中国疾病预防控制中心(2017)
$p$	26	潜伏者变为染病者的比例	中国疾病预防控制中心(2017)
$\gamma$	20	染病者的恢复率	中国疾病预防控制中心(2017)
$k$	8	染病者排放到环境中的病毒的速率	假设
$\tau$	1.6	环境中病毒的失效率	假设

[1]	Wei Fengying, Lin Qingteng. Extinction and Distribution for an SIQS Epidemic Model with Quarantined-Adjusted Incidence [J]. Acta mathematica scientia,Series A, 2017, 37(6): 1148-1161.
[2]	Meng Xiaoying. Analysis of a Stochastic Delayed Epidemic Model with a Non-Monotonic Incidence Rate [J]. Acta mathematica scientia,Series A, 2017, 37(6): 1162-1175.
[3]	Wei Aiju, Zhang Xinjian, Wang Junyi, Li Kezan. Dynamics Analysis of an Ebola Epidemic Model [J]. Acta mathematica scientia,Series A, 2017, 37(3): 577-592.
[4]	Wang Heyuan. The Dynamical Behaviors and the Numerical Simulation of a Five-Mode Lorenz-Like System of the MHD Equations for a Two-Dimensional Incompressible Fluid on a Torus [J]. Acta mathematica scientia,Series A, 2017, 37(1): 199-216.
[5]	YE Hui, CAI Dong-Han. Global Attractivity in a Periodic Nicholson's Blowflies Model [J]. Acta mathematica scientia,Series A, 2013, 33(6): 1013-1021.
[6]	LI Yu-Huan, ZHOU Jun, MU Chun-Lai. Stability Analysis for a Predator-Prey System with Nonlocal Delayed Reaction-diffusion Equations [J]. Acta mathematica scientia,Series A, 2012, 32(3): 475-488.
[7]	CUI Cheng, WANG Xiao, GAO Fei, LIU An-Ping. Global Stability of Periodic Solution and Almost Periodic Solution of Price Cooperating Model with Time Lag [J]. Acta mathematica scientia,Series A, 2011, 31(6): 1718-1729.
[8]	LI An, SONG Xin-Yu, WANG Zhi-Xiang. Practical Stability of Nonlinear Differential Equations Relative to Initial Time Difference via Perturbing Lyapunov Functions [J]. Acta mathematica scientia,Series A, 2011, 31(2): 351-359.
[9]	XU Yong-Chun, HE Xin-Feng, HOU Zhi-Bin, HE Zhen. Generalized Projection Methods for Noor Variational Inequalities in Banach Spaces [J]. Acta mathematica scientia,Series A, 2010, 30(3): 808-817.
[10]	Wu Shiliang ;Li Wantong. Stability and Traveling Fronts in Lotka-Volterra Cooperation Model with Stage Structure [J]. Acta mathematica scientia,Series A, 2008, 28(3): 454-464.
[11]	Wang Yuquan; Liu Laifu. On the Dynamics of a Food Chain with Monod-Haldane Functional Response [J]. Acta mathematica scientia,Series A, 2007, 27(1): 79-089.
[12]	Wang Yuquan ;Jing Zhujun. Global Qualitative Analysis of a Food Chain Model [J]. Acta mathematica scientia,Series A, 2006, 26(3): 410-420.
[13]	Chen Fulai ;Wen Xianzhang. Impulsive Asymptoticity for Impulsive Functional Differential Equation [J]. Acta mathematica scientia,Series A, 2006, 26(2): 287-296.
[14]	ZHANG Yu, WANG Chun-Yan, SUN Ji-Chao. Stability of Impulsive Differential Equations with Impulses at Variable Times [J]. Acta mathematica scientia,Series A, 2005, 25(6): 777-783.
[15]	CENG Zhi-Gang, LIAO Xiao-Cuan. Global Stability for Neural Networks with Unbouned Time Varying Delays [J]. Acta mathematica scientia,Series A, 2005, 25(5): 621-626.

Global Stability of a Measles Epidemic Model with Partial Immunity and Environmental Transmission

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 4

References 13

Related Articles 15

Metrics

Comments

Recommended 0