具有变时滞的神经型Hopfield神经网络的全局吸引子研究

Abstract

Abstract:

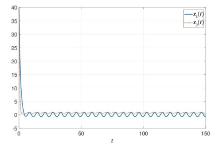
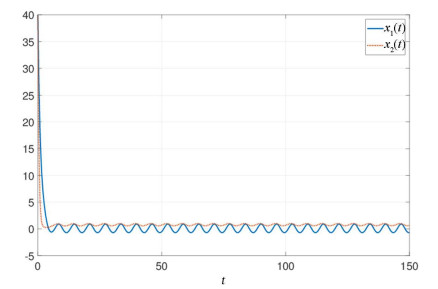
This paper deals with the asymptotic properties of a class of nonlinear and nonautonomous neutral type Hopfield neural networks with time-varying delays. By applying the property of nonnegative matrix and an integral inequality, some sufficient conditions are derived to ensure the existence of the global attracting set and the stability in a Lagrange sense for the considered system. Finally, an example is given to demonstrate the effectiveness of our theoretical result.

Key words: Neutral-type Hopfield neural networks, Time-varying delays, Lagrange stability, Global attracting set, Integral inequality

CLC Number:

O193

Qinghua Zhou,Li Wan,Jie Liu. Global Attracting Set for Neutral Type Hopfield Neural Networks with Time-Varying Delays[J].Acta mathematica scientia,Series A, 2019, 39(4): 823-831.

Trendmd

Figures/Tables 1

References 37

1	Huang Y , Yang X S . Hyperchaos and bifurcation in a new class of four-dimensional Hopfield neural networks. Neurocomputing, 2006, 69: 1787- 1795 doi: 10.1016/j.neucom.2005.11.001
2	He W , Cao J . Stability and bifurcation of a class of discrete-time neural networks. Appl Math Model, 2007, 31: 2111- 2122 doi: 10.1016/j.apm.2006.08.006
3	Huang W , Huang Y . Chaos of a new class of Hopfield neural networks. Appl Math Comput, 2008, 206: 1- 11
4	Kaslik E , Balint St . Bifurcation analysis for a discrete-time Hopfield neural network of two neurons with two delays and self-connections. Chaos Soliton Fract, 2009, 39: 83- 91 doi: 10.1016/j.chaos.2007.01.126
5	Zheng P S , Tang W S , Zhang J X . Some novel double-scroll chaotic attractors in Hopfield networks. Neurocomputing, 2010, 73: 2280- 2285 doi: 10.1016/j.neucom.2010.02.015
6	Marichal R L , Gonzalez E J , Marichal G N . Hopf bifurcation stability in Hopfield neural networks. Neural Netw, 2012, 36: 51- 58 doi: 10.1016/j.neunet.2012.09.007
7	Mazrooei-Sebdani R , Farjami S . Bifurcations and chaos in a discrete-time-delayed Hopfield neural network with rings tructures and different internal decays. Neurocomputing, 2013, 99: 154- 162 doi: 10.1016/j.neucom.2012.06.007
8	Akhmet M , Fen M . Generation of cyclic/toroidal chaos by Hopfield neural networks. Neurocomputing, 2014, 145: 230- 239 doi: 10.1016/j.neucom.2014.05.038
9	Mazrooei-Sebdani R , Farjami S . On a discrete-time-delayed Hopfield neural network with ring structures and different internal decays: Bifurcations analysis and chaotic behavior. Neurocomputing, 2015, 151: 188- 195 doi: 10.1016/j.neucom.2014.06.079
10	Wang Q , Fang Y Y , Li H , Su L J , Dai B X . Anti-periodic solutions for high-order Hopfield neural networks with impulses. Neurocomputing, 2014, 138: 339- 346 doi: 10.1016/j.neucom.2014.01.028
11	Yang L , Li Y K . Existence and exponential stability of periodic solution for stochastic Hopfield neural networks on time scales. Neurocomputing, 2015, 167: 543- 550 doi: 10.1016/j.neucom.2015.04.038
12	Wang C . Piecewise pseudo-almost periodic solution for impulsive non-autonomous high-order Hopfield neural networks with variable delays. Neurocomputing, 2016, 171: 1291- 1301 doi: 10.1016/j.neucom.2015.07.054
13	Kaslik E , Sivasundaram S . Multistability in impulsive hybrid Hopfield neural networks with distributed delays. Nonlinear Anal-Real, 2011, 12: 1640- 1649 doi: 10.1016/j.nonrwa.2010.10.018
14	Park M J , Kwon O M , Park J H , Lee S M . Simplified stability criteria for fuzzy Markovian jumping Hopfield neural networks of neutral type with interval time-varying delays. Expert Syst Appl, 2012, 39: 5625- 5633 doi: 10.1016/j.eswa.2011.11.055
15	Wang H , Yu Y G , Wen G G . Stability analysis of fractional-order Hopfield neural networks with time delays. Neural Netw, 2014, 55: 98- 109 doi: 10.1016/j.neunet.2014.03.012
16	Wang H , Yu Y G , Wen G G , et al. Global stability analysis of fractional-order Hopfield neural networks with time delay. Neurocomputing, 2015, 154: 15- 23 doi: 10.1016/j.neucom.2014.12.031
17	Dharani S , Rakkiyappan R , Cao J D . New delay-dependent stability criteria for switched Hopfield neural networks of neutral type with additive time-varying delay components. Neurocomputing, 2015, 151: 827- 834 doi: 10.1016/j.neucom.2014.10.014
18	Song B , Zhang Y , Shu Z , Hu F N . Stability analysis of Hopfield neural networks perturbed by Poisson noises. Neurocomputing, 2016, 196: 53- 58 doi: 10.1016/j.neucom.2016.02.034
19	Zhang S , Yu Y G , Wang Q . Stability analysis of fractional-order Hopfield neural networks with discontinuous activation functions. Neurocomputing, 2016, 171: 1075- 1084 doi: 10.1016/j.neucom.2015.07.077
20	Liao X X , Luo Q , Zeng Z G . Positive invariant and global exponential attractive sets of neural networks with time-varying delays. Neurovomputing, 2008, 71: 513- 518 doi: 10.1016/j.neucom.2007.07.017
21	Crauel H , Debussche A , Flandoli F . Random attractors. J Dynam Differ Equ, 1995, 9: 307- 341
22	Kloeden P , Stonier D J . Cocycle attractors in nonautonomously perturbed dif- ferential equations. Dynam Continuous Discrete Impulsive Systems, 1998, 4: 211- 226
23	Cheban D N . Dissipative functional differential equations. Bull Acad Sci Rep Moldova Mat, 1991, 2 (5): 3- 12
24	Caraballo T , Marn-Rubio P , Valero J . Autonomous and non-autonomous attractors for differential equations with delays. J Differ Equ, 2005, 208: 9- 41 doi: 10.1016/j.jde.2003.09.008
25	Caraballo T , Kloeden P E , Real J . Pullback and forward attractors for a damped wave equation with delays. Stoch Dyn, 2004, 4: 405- 423 doi: 10.1142/S0219493704001139
26	Caraballo T , Real J . Attractors for 2D-Navier-Stokes models with delays. J Differ Equ, 2004, 205: 271- 297 doi: 10.1016/j.jde.2004.04.012
27	Zhang L X , Boukas E K , Haidar A . Delay-range-dependent control synthesis for time-delay systems with actuator saturation. Automatica, 2008, 44: 2691- 2695 doi: 10.1016/j.automatica.2008.03.009
28	Xu D Y . Integro-differential equations and delay integral inequalities. Tohoku Math J, 1992, 44: 365- 378 doi: 10.2748/tmj/1178227303
29	Zhao H Y . Invariant set and attractor of nonautonomous functional differential systems. J Math Anal Appl, 2003, 282: 437- 443 doi: 10.1016/S0022-247X(02)00370-0
30	Xu D Y , Yang Z C . Attracting and invariant sets for a class of implusive functional defferential equations. J Math Anal Appl, 2007, 329: 1036- 1044 doi: 10.1016/j.jmaa.2006.05.072
31	Tian H . The exponential asymtotic stability of singularly perturbed delay differential equations with a bounded lag. J Math Anal Appl, 2002, 270: 143- 149 doi: 10.1016/S0022-247X(02)00056-2
32	Xu D Y , Yang Z G . Exponential stability of nonlinear implusive neutral differential equations with delays. Nonlinear Anal Theory Methods Appl, 2007, 67: 1426- 1439 doi: 10.1016/j.na.2006.07.043
33	Zhu W . Global exponential stability of impulisive reaction-diffusion equation with variable delays. Appl Math Comput, 2008, 205: 362- 369
34	Teng L Y , Xu D Y . Global attracting set for non-autonomous neutral networks with distributed delays. Neurocomputing, 2012, 94: 64- 67 doi: 10.1016/j.neucom.2012.04.020
35	Liu L , Zhu Q X , Feng L C . Lagrange stability for delayed recurrent neural networks with Markovian switching based on stochastic vector Halandy inequalities. Neurocomputing, 2018, 275: 1614- 1621 doi: 10.1016/j.neucom.2017.10.006
36	Popa A C . Global exponential stability of neutral-type octonion-valued neural networks with time-varying delays. Neurocomputing, 2018, 309: 117- 133 doi: 10.1016/j.neucom.2018.05.004
37	LaSalle J P. The Stability of Dynamical System. Philadelphia: SIAM, 1976

[1]	Xi Boyan, Qi Feng. Some Integral Inequalities of Hermite-Hadamard Type for s-Logarithmically Convex Functions [J]. Acta mathematica scientia,Series A, 2015, 35(3): 515-524.
[2]	WU Yu, TANG Min, ZHOU Cha-Jin. A New Weakly Singular Wendroff Integral Inequalities [J]. Acta mathematica scientia,Series A, 2014, 34(6): 1465-1473.
[3]	LIU Qiong. A Integral Inequality with Multi-parameters and the Kernel of Hyperbolic Cotangent Function [J]. Acta mathematica scientia,Series A, 2014, 34(4): 851-858.
[4]	LIU Qiong, LONG Shun-Chao. A Generalization of Hilbert-type Integral Inequality [J]. Acta mathematica scientia,Series A, 2014, 34(1): 179-185.
[5]	ZHANG Yu-Tian, LUO Qi. Global Exponential Stability of Impulsive Reaction-Diffusion Cellular Neural Networks with Time-Varying Delays and Neumann Boundary Condition [J]. Acta mathematica scientia,Series A, 2013, 33(4): 777-786.
[6]	KUANG Ji-Chang. Hilbert-type Integral Inequalities in Normed Linear Spaces [J]. Acta mathematica scientia,Series A, 2013, 33(1): 1-5.
[7]	HONG Yong. On a Multiple Hardy-type Integral Inequality with a Best Constant Factor and Its Application [J]. Acta mathematica scientia,Series A, 2011, 31(6): 1586-1591.
[8]	LI Jun-Min. Adaptive Iterative Learning Control for Nonlinearly Parameterized Systems with Time-Varying Delays [J]. Acta mathematica scientia,Series A, 2011, 31(3): 682-690.
[9]	XIN Dong-Mei, YANG Bi-Cheng. On a New Extension of a Hilbert-type Integral Inequality [J]. Acta mathematica scientia,Series A, 2010, 30(6): 1648-1653.
[10]	LU Sheng-Jie, TAO Xiang-Xing. Multiple Unsymmetrical Core Hardy-Hilbert's Integral Inequalities [J]. Acta mathematica scientia,Series A, 2009, 29(3): 597-606.
[11]	Gao Hongya; Hou Lanru. Two-Weight Integral Inequalities for Conjugate ${\cal A}$-Harmonic Tensors [J]. Acta mathematica scientia,Series A, 2008, 28(2): 341-348.
[12]	Li Juan. Two-weight Integral Inequalities for Differential Forms [J]. Acta mathematica scientia,Series A, 2007, 27(3): 515-523.

Global Attracting Set for Neutral Type Hopfield Neural Networks with Time-Varying Delays

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 1

References 37

Related Articles 12

Metrics

Comments

Recommended 3