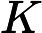数学物理学报(英文版) ›› 2021, Vol. 41 ›› Issue (6): 1938-1948.doi: 10.1007/s10473-021-0610-6
LAPLACE TRANSFORMS FOR ANALYTIC FUNCTIONS IN TUBULAR DOMAINS
邓冠铁, 付倩, 曹辉
- Guantie DENG, Qian FU, Hui CAO
LAPLACE TRANSFORMS FOR ANALYTIC FUNCTIONS IN TUBULAR DOMAINS
Guantie DENG, Qian FU, Hui CAO
- Guantie DENG, Qian FU, Hui CAO
摘要: Assume that and that is a connected nonempty open set in , and that is the vector space of all holomorphic functions in the tubular domains such that for any compact set
so is a Fréchet space with the Heine-Borel property, its topology is induced by a complete invariant metric, is not locally bounded, and hence is not normal. Furthermore, if , then the element of can be written as a Laplace transform of some function .
中图分类号:
- 42B30