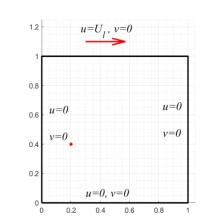
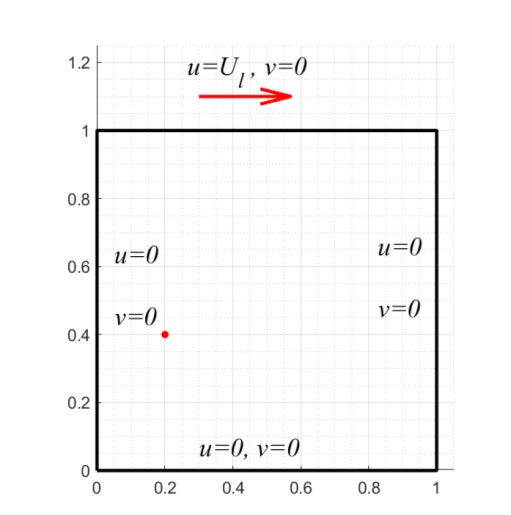
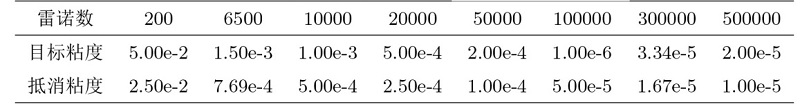
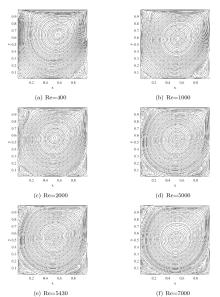
Acta mathematica scientia,Series A ›› 2024, Vol. 44 ›› Issue (5): 1216-1229.
Previous Articles Next Articles
Research on High Reynolds Number Flow Using MRT-LBM with Viscosity Counteracting
Zhang Zongning1,Zhang Qiaoling2,*( ),Jing Hefang3,Shen Qixia1
),Jing Hefang3,Shen Qixia1
- 1Department of Basic Courses, Zhengzhou University of Science and Technology, Zhengzhou 450064
2Department of Teaching and Research of Basic Courses, Guangdong Technology College, Guangdong Zhaoqing 526100
3School of Civil Engineering, North Minzu University, Yinchuan 750021
-
Received:2023-12-29Revised:2024-04-28Online:2024-10-26Published:2024-10-16 -
Supported by:NSFC(11861003);NSFC(11761005);Ningxia Natural Science Foundation(2023AAC02049);Ningxia Natural Science Foundation(2022AAC02004);Key scientific research project of Henan(24B110020)
CLC Number:
- O357.5
Cite this article
Zhang Zongning, Zhang Qiaoling, Jing Hefang, Shen Qixia. Research on High Reynolds Number Flow Using MRT-LBM with Viscosity Counteracting[J].Acta mathematica scientia,Series A, 2024, 44(5): 1216-1229.
share this article
| [1] |
Hou S, Zou Q, Chen S, et al. Simulation of cavity flow by the lattice Boltzmann method. Journal of Computational Physics, 1994, 118(2): 329-347
doi: 10.1006/jcph.1995.1103 |
| [2] | Feldman Y, Gelfgat A Y. Oscillatory instability of a three-dimensional lid-driven flow in a cube. Physics of Fluids, 2010, 22(9): 093602 |
| [3] | Shan X W. The mathematical structure of the lattices of the lattice Boltzmann method. Journal of Computational Science, 2016, 17(2): 475-481 |
| [4] | Somers J A. Direct simulation of fluid flow with cellular automata and the lattice-Boltzmann equation. Applied Scientific Research, 1993, 51(1/2): 127-133 |
| [5] | 张宗宁, 李春光, 董建强. 变系数广义 KdV-Burgers 方程的格子 Boltzmann 模型. 数学物理学报, 2021, 41A(5): 1283-1295 |
| Zhang Z Z, Li C G, Dong J Q. General Propagation Lattice Boltzmann Model for a Variable-Coefficient Compound KdV-Burgers Equation. Acta Math Sci, 2021, 41A(5): 1283-1295 | |
| [6] | Chen S Y, Doolen G D. Lattice Boltzmann method for fluid flows. Annu Rev Fluid Mech, 1998, 30(1): 329-364 |
| [7] | Boghosian B M, Boon J P. Lattice Boltzmann and nonextensive diffusion. Europhysics News, 2005, 36(6): 192-194 |
| [8] | Qian Y H, D'Humieres D, Lallemand P. Lattice BGK Models for the Navier-Stokes Equations. Europhysics Letters, 1992, 17(6): 479-484 |
| [9] | He X, Doolen G. Lattice Boltzmann method on curvilinear coordinates system: Flow around a circular cylinder. Journal of Computational Physics, 1997, 134(2): 306-315 |
| [10] | Sterling J D, Chen S. Stability analysis of lattice Boltzmann methods. Journal of Computational Physics, 1996, 123(1): 196-206 |
| [11] | D'Humieres D. Generalized lattice Boltzmann equations. Rarefied Gas Dynamics-Theory and Simulations, 1994: 450-458 |
| [12] | Luo L S, Liao W, Chen X, et al. Numerics of the lattice Boltzmann method: Effects of collision models on the lattice Boltzmann simulations. Physical Review E, 2011, 83(5): 056710 |
| [13] | Chai Z H, Shi B C. Multiple-relaxation-time lattice Boltzmann method for the Navier-Stokes and nonlinear conveection-diffusion equations: Modeling, analysis and elements. Physical Review E, 2020, 102(2): 023306 |
| [14] | Luo L S, Krafczyk M, Shyy W. Lattice Boltzmann Method for Computational Fluid Dynamics. John Wiley & Sons, Ltd, 2010 |
| [15] | Yu H D, Luo L S, Girimaji S S. LES of turbulent square jet flow using an MRT lattice Boltzmann model. Comput Fluids, 2006, 35: 957-965 |
| [16] | Jing H F, Cai Y J, Wang W H, et al. Investigation of open channel flow with unsubmerged rigid vegetation by the lattice Boltzmann method. Journal of Hydrodynamics, 2020, 4: 771-783 |
| [17] |
张巧玲, 景何仿. 三维曲面腔顶盖驱动流的 MRT-LBM 研究. 计算物理, 2022, 39(4): 427-439
doi: 10.19596/j.cnki.1001-246x.8447 |
|
Zhang Q L, Jing H F. Flow patterns in three-dimensional lid-driven cavities with curved boundary : MRT-LBM study. Chinese Journal of Computional Physics, 2022, 39(4): 427-439
doi: 10.19596/j.cnki.1001-246x.8447 |
|
| [18] | Zhang K, Feng X F, Jing H F, Jiang Y L. An improved MRT-LBM and investigation to the transition and periodicity of 2D lid-driven cavity flow with high Reynolds numbers. Chinese Journal of Physics, 2023, 84: 51-65 |
| [19] | Das S S, Das P K. Fluid flow in a cavity driven by an oscillating lid-A simulation by lattice Boltzmann method. European Journal of Mechanics-B/Fluids, 2013, 39(3): 59-70 |
| [20] | Premnath K N, Pattison M J, Banerjee S. Generalized lattice Boltzmann equation with forcing term for computation of wall-bounded turbulent flows. Physical Review E, 2009, 79(2): 026703 |
| [21] | Cheng Y G, Zhang H. A viscosity counteracting approach in the lattice Boltzmann BGK model for low viscosity flow: Preliminary verification. Computers & Mathematics with Applications, 2011, 61(12): 3690-3702 |
| [22] | Zhang C Z, Cheng Y G, Huang S, et al. Improving the stability of the multiple-relaxation-time lattice Boltzmann method by a viscosity counteracting approach. Advances in Applied Mathematics & Mechanics, 2016, 8(1): 37-51 |
| [23] | Lallemand P, Luo L S. Theory of the lattice Boltzmann method: dispersion, dissipation, isotropy, galilean invariance, and stability. Physical review E, 2000, 61(6): 6546-6562 |
| [24] | Cheng Y G, Li J P. Introducing unsteady non-uniform source terms into the lattice Boltzmann model. International Journal for Numerical Methods in Fluids, 2008, 56(6): 629-641 |
| [25] | Ghia U, Ghia K N, Shin C T. High-Re solutions for incompressible flow using the Navier-Stokes equations and a multigrid method. Journal of Computational Physics, 1982, 48(3): 387-411 |
| [26] | Erturk E, Corke T C, Gokcol C. Numerical solutions of 2-D steady incompressible driven cavity flow at high Reynolds numbers. Int J Numer Meth Fluid, 2005, 48(7): 747-774 |
| [27] | Guo Z L, Zheng C G, Shi B C. Non-equilibrium extrapolation method for velocity and pressure boundary conditions in the lattice Boltzmann method. Chinese Physics, 11(4): 366-374 |
| No related articles found! |
|