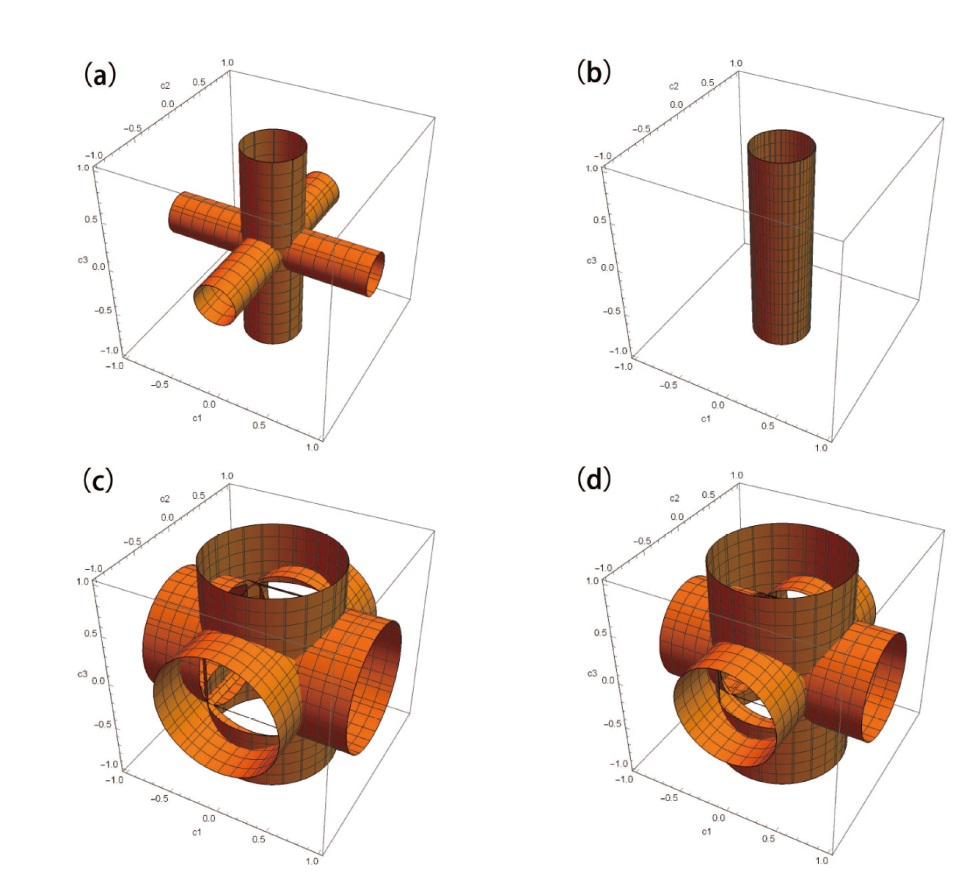
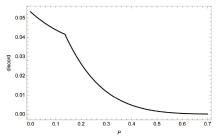
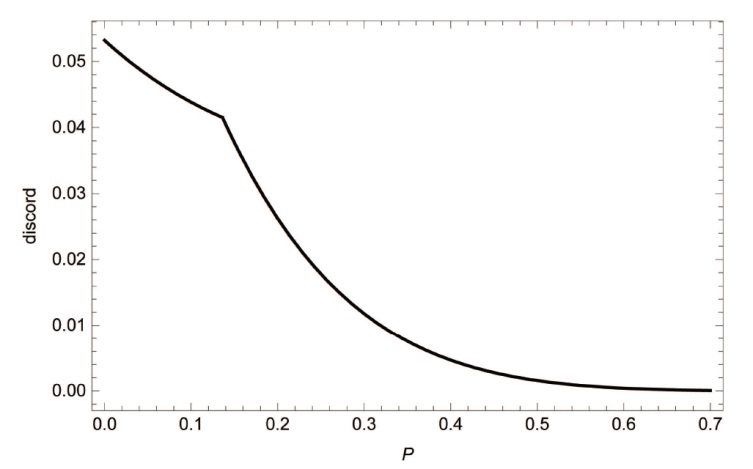
Acta mathematica scientia,Series A ›› 2024, Vol. 44 ›› Issue (5): 1144-1152.
Previous Articles Next Articles
Geometric Discord for a Class of Three-Qubit X States
Wei Jianing1( ),Duan Zhoubo1,*(
),Duan Zhoubo1,*( ),Zhang Jun1,2(
),Zhang Jun1,2( )
)
- 1School of Mathematics, Taiyuan University of Technology, Taiyuan 030024
2College of Computer Science and Technology (College of Data Science), Taiyuan University of Technology, Taiyuan 030024
-
Received:2022-10-26Revised:2024-04-29Online:2024-10-26Published:2024-10-16 -
Supported by:National Natural Science Foundation of China(11771011);Natural Science Foundation of Shanxi Province(201801D221032);Natural Science Foundation of Shanxi Province(202303021211068);Shanxi Scholarship Council of China(2023-081)
CLC Number:
- O177
Cite this article
Wei Jianing, Duan Zhoubo, Zhang Jun. Geometric Discord for a Class of Three-Qubit X States[J].Acta mathematica scientia,Series A, 2024, 44(5): 1144-1152.
share this article
| [1] | Ollivier H, Zurek W H. Quantum discord: A measure of the quantumness of correlations. Physical Review Letters, 2001, 88(1): 017901 |
| [2] | Henderson L, Vedral V. Classical, quantum and total correlations. Journal of Physics A: Mathematical and General, 2001, 34(35): 6899 |
| [3] | Lanyon B P, Barbieri M, Almeida M P, et al. Experimental quantum computing without entanglement. Physical Review Letters, 2008, 101(20): 200501 |
| [4] | Merali Z. The power of discord: Physicists have always thought quantum computing is hard because quantum states are incredibly fragile. But could noise and messiness actually help things along? Nature, 2011, 474(7349): 24-27 |
| [5] | Li N, Luo S. Total versus quantum correlations in quantum states. Physical Review A, 2007, 76(3): 032327 |
| [6] | Mazzola L, Piilo J, Maniscalco S. Sudden transition between classical and quantum decoherence. Physical Review Letters, 2010, 104(20): 200401 |
| [7] | Niset J, Cerf N J. Multipartite nonlocality without entanglement in many dimensions. Physical Review A, 2006, 74(5): 052103 |
| [8] | Werlang T, Souza S, Fanchini F F, et al. Robustness of quantum discord to sudden death. Physical Review A, 2009, 80(2): 024103 |
| [9] | Sarandy M S. Classical correlation and quantum discord in critical systems. Physical Review A, 2009, 80(2): 022108 |
| [10] | Dakič B, Lipp Y O, Ma X, et al. Quantum discord as resource for remote state preparation. Nature Physics, 2012, 8(9): 666-670 |
| [11] | Dillenschneider R. Quantum discord and quantum phase transition in spin chains. Physical Review B, 2008, 78(22): 224413 |
| [12] | Pirandola S. Quantum discord as a resource for quantum cryptography. Scientific Reports, 2014, 4(1): 06956 |
| [13] | Rulli C C, Sarandy M S. Global quantum discord in multipartite systems. Physical Review A, 2011, 84(4): 042109 |
| [14] | Luo S, Fu S. Geometric measure of quantum discord. Physical Review A, 2010, 82(3): 034302 |
| [15] | Radhakrishnan C, Laurière M, Byrnes T. Multipartite generalization of quantum discord. Physical Review Letters, 2020, 124(11): 110401 |
| [16] | Zhu C L, Hu B, Li B, et al. Geometric discord for multiqubit systems. Quantum Information Processing, 2022, 21(7): 1-15 |
| [17] | Maziero J, Celeri L C, Serra R M, et al. Classical and quantum correlations under decoherence. Physical Review A, 2009, 80(4): 044102 |
| [18] | Yu T, Eberly J H. Quantum open system theory: Bipartite aspects. Physical Review Letters, 2006, 97(14): 140403 |
| [1] | Chai Mengcen, Dai Yuxia. Freely Quasiconformal Mappings in Quasiconvex Metric Spaces [J]. Acta mathematica scientia,Series A, 2024, 44(5): 1127-1135. |
| [2] | Ding Xuanhao, Shao Changhui, Li Yongning. The Product of Volterra Operator and Toeplitz Operator [J]. Acta mathematica scientia,Series A, 2024, 44(4): 829-836. |
| [3] | Chen Xi, Wang Zhengping. Constrained Minimizers of Nonlinear S-P Equations with Dirac Potentials [J]. Acta mathematica scientia,Series A, 2024, 44(4): 907-913. |
| [4] | Hong Yong, Zhao Qian. Parameter Conditions for Constructing Bounded Discrete Operators with Super-Homogeneous Kernel and Estimation of the Operator Norm [J]. Acta mathematica scientia,Series A, 2024, 44(4): 837-846. |
| [5] | Dong Jianxiang. Hankel Operators on Vector-Valued Bergman Space with Exponential Type Weights [J]. Acta mathematica scientia,Series A, 2024, 44(3): 513-524. |
| [6] | Wang Panxing, Liang Yuxia, Pang Songyue. Numerical Range of the Complex Volterra Operator on Hardy Hilbert Space [J]. Acta mathematica scientia,Series A, 2024, 44(2): 276-285. |
| [7] | Zhou Yue, Yang Yan. Uncertainty Principles of Fractional Fourier Transform [J]. Acta mathematica scientia,Series A, 2024, 44(2): 257-264. |
| [8] | Zheng Lanling, Ding Huisheng. Almost Automorphy for a Class of Delay Differential Equations with Non-densely Defined Operators on Banach Spaces [J]. Acta mathematica scientia,Series A, 2024, 44(2): 361-375. |
| [9] | Chen Mingchao, Xue Yanfang. Multiple Solutions for a Class of Quasilinear Schrödinger Equations with a Perturbed Term [J]. Acta mathematica scientia,Series A, 2024, 44(2): 417-428. |
| [10] | Jian-Xiang DONG. Hankel Operators on vector-valued Bergman space with exponential type weights [J]. Acta mathematica scientia,Series A, 0, (): 0-0. |
| [11] | Jian Weigang, Long Wei. Tauberian Theorem for Asymptotically Periodic Functions and Its Application to Abstract Cauchy Problems [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1699-1709. |
| [12] | Li Renhua, Wang Zhengping. Normalized Solution of Fractional Schrödinger-Poisson Equations with Coercive Potential [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1723-1730. |
| [13] | Zhou Yinggao, Li Zhouxin. An Application of Linking Theorem to Degenerative Elliptic Equations [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1759-1773. |
| [14] | Pan Lingrong, Wang Yuanheng. The Strong Convergence Theorem of Iterative Algorithms for the Fixed Point Problem, a System of Variational Inequalities, and a Split Equilibrium Problem in Hilbert Spaces [J]. Acta mathematica scientia,Series A, 2023, 43(6): 1880-1896. |
| [15] | Ding Xuanhao,Hou Lin,Li Yongning. The Reproducing Kernel of Bergman Space and the Eigenvectors of Toeplitz Operator [J]. Acta mathematica scientia,Series A, 2023, 43(5): 1333-1340. |
|