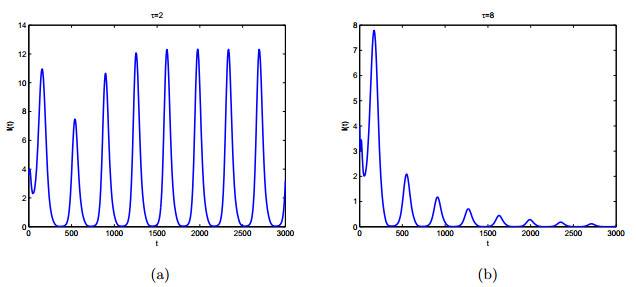
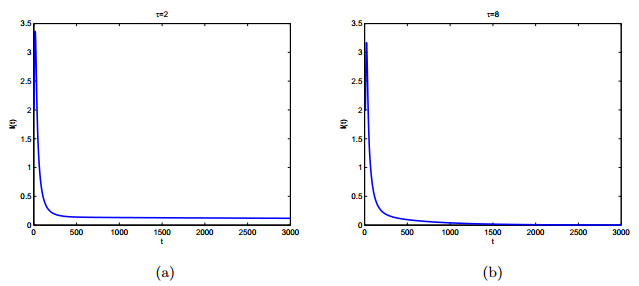
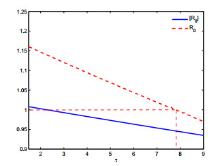
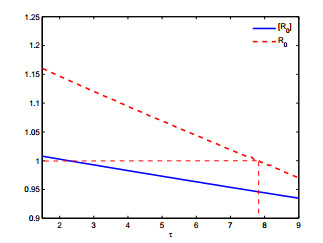
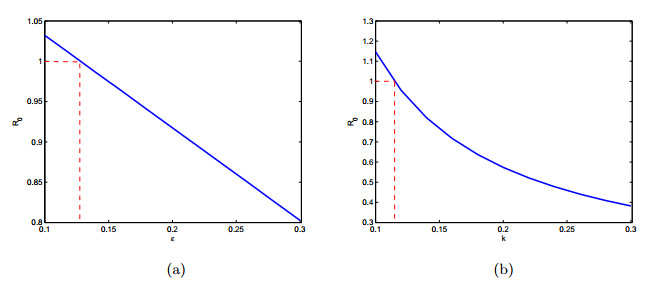
Acta mathematica scientia,Series A ›› 2022, Vol. 42 ›› Issue (3): 851-866.
Previous Articles Next Articles
Dynamics of an Anthrax Epidemiological Model with Time Delay and Seasonality
Tailei Zhang1,Junli Liu2,*( ),Mengjie Han2
),Mengjie Han2
- 1 School of Science, Chang'an University, Xi'an 710064
2 School of Science, Xi'an Polytechnic University, Xi'an 710048
-
Received:2021-08-23Online:2022-06-26Published:2022-05-09 -
Contact:Junli Liu E-mail:jlliu2008@126.com -
Supported by:the NSFC(11801431);the Natural Science Basic Research Plan in Shaanxi Province(2021JM-445);the Natural Science Basic Research Plan in Shaanxi Province(2022JM-023)
CLC Number:
- O175.1
Cite this article
Tailei Zhang,Junli Liu,Mengjie Han. Dynamics of an Anthrax Epidemiological Model with Time Delay and Seasonality[J].Acta mathematica scientia,Series A, 2022, 42(3): 851-866.
share this article
| 1 | CDC: Use of anthrax vaccine in the United States recommendations of the Advisory Committee on Immunization Practices (ACIP), 2009. Morb Mort Wkly Rep, 2010, 59(6): 1-36 |
| 2 | Survely A N , Kvasnicka B , Torell R . Anthrax: a guide for livestock producers, Cattle producer's Library CL613. Western Beef Resource Committee, 2001, 613, 1- 3 |
| 3 |
Hahn B D , Furniss P R . A deterministic model of an anthrax epizootic: threshold results. Ecol Model, 1983, 20, 233- 241
doi: 10.1016/0304-3800(83)90009-1 |
| 4 |
Friedman A , Yakubu A A . Anthrax epizootic and migration: Persistence or extinction. Math Biosci, 2013, 241, 137- 144
doi: 10.1016/j.mbs.2012.10.004 |
| 5 |
Mushayabasa S , Marijani T , Masocha M . Dynamical analysis and control strategies in modeling anthrax. Comp Appl Math, 2017, 36, 1333- 1348
doi: 10.1007/s40314-015-0297-1 |
| 6 |
Mushayabasa S . Dynamics of an anthrax model with distributed delay. Acta Appl Math, 2016, 144, 77- 86
doi: 10.1007/s10440-016-0040-y |
| 7 |
Saad-Roy C M , van den Driessche P , Yakubu A A . A mathematical model of anthrax transmission in animal populations. Bull Math Biol, 2017, 79, 303- 324
doi: 10.1007/s11538-016-0238-1 |
| 8 | Lindeque P , Turnbull P . Ecology and epidemiology of anthrax in the Etosha National Park, Namibia. Onderstepoort J Vet, 1994, 61 (1): 71- 83 |
| 9 | Lembo T , Hampson K , Authy H , et al. Serologic surveillance of anthrax in the Serengeti ecosystem, Tanzania, 1996-2009. Emerg Infect Dis, 2001, 17, 387- 394 |
| 10 |
Van den Driessche P , Yakubu A A . Disease extinction versus persistence in discrete-time epidemic models. Bull Math Biol, 2019, 81, 4412- 4446
doi: 10.1007/s11538-018-0426-2 |
| 11 | Lou Y J , Zhao X Q . Threshold dynamics in a time-delayed periodic SIS epidemic model. Discrete Contin Dyn Syst Ser B, 2009, 12, 169- 186 |
| 12 | Hale J K , Verduyn Lunel S M . Introduction to Functional Differential Equations. New York: Springer, 1993 |
| 13 | Smith H L. Monotone Dynamical Systems: An Introduction to the Theory of Competitive and Cooperative Systems. Providence, RI: American Mathematical Society, 1995 |
| 14 |
Zhao X Q . Basic reproduction ratios for periodic compartmental models with time delay. J Dyn Differ Equ, 2017, 29, 67- 82
doi: 10.1007/s10884-015-9425-2 |
| 15 |
Walter W . On strongly monotone flows. Ann Polon Math, 1997, 66, 269- 274
doi: 10.4064/ap-66-1-269-274 |
| 16 |
Liang X , Zhao X Q . Asymptotic speeds of spread and traveling waves for monotone semiflows with applications. Comm Pure Appl Math, 2007, 60, 1- 40
doi: 10.1002/cpa.20154 |
| 17 |
Wang X N , Zhao X Q . Dynamics of a time-delayed Lyme disease model with seasonality. SIAM J Appl Dyn Syst, 2017, 16, 853- 881
doi: 10.1137/16M1087916 |
| 18 | Zhao X Q . Dynamical Systems in Population Biology. New York: Springer, 2017 |
| 19 |
Magal P , Zhao X Q . Global attractors and steady states for uniformly persistent dynamical systems. SIAM J Math Anal, 2005, 37, 251- 275
doi: 10.1137/S0036141003439173 |
| 20 |
Yuan Y , Zhao X Q . Global stability for non-monotone delay equations (with application to a model of blood cell production). J Differ Equ, 2012, 252, 2189- 2209
doi: 10.1016/j.jde.2011.08.026 |
| 21 | Lewerin S S, Elvander M, Westermark T, et al. Anthrax outbreak in a Swedish beef cattle herd-1st case in 27 years: Case report. Acta Vet Scand, 2010, 52, Article number: 7 |
| 22 |
Liang X , Zhang L , Zhao X Q . Basic reproduction ratios for periodic abstract functional differential equations (with application to a spatial model for Lyme disease). J Dyn Differ Equ, 2019, 31, 1247- 1278
doi: 10.1007/s10884-017-9601-7 |
| 23 | World Health Organization . Anthrax in Humans and Aanimals. 4th edn Geneva: World Health Organization, 2008: 29 |
| [1] | Xiaodong Kang,Hongxia Fan. Approximate Controllability for a Class of Second-Order Evolution Equations with Instantaneous Impulse [J]. Acta mathematica scientia,Series A, 2023, 43(2): 421-432. |
| [2] | Zhenxiang Hu,Linfei Nie. Analysis of a Reaction-Diffusion Epidemic Model with Horizontal Transmission and Environmental Transmission [J]. Acta mathematica scientia,Series A, 2022, 42(6): 1849-1860. |
| [3] | Yage Tong,Kaisu Wu. Spectral Analysis of the Main Operator of Rotenberg Equation with Time Delay Under Nonsmooth Boundary Conditions [J]. Acta mathematica scientia,Series A, 2022, 42(5): 1320-1331. |
| [4] | Kai Wang,Hongyong Zhao. Traveling Wave of a Reaction-Diffusion Dengue Epidemic Model with Time Delays [J]. Acta mathematica scientia,Series A, 2022, 42(4): 1209-1226. |
| [5] | Daoxiang Zhang,Ben Li,Dandan Chen,Yating Lin,Xinmei Wang. Hopf Bifurcation for a Fractional Differential-Algebraic Predator-Prey System with Time Delay and Economic Profit [J]. Acta mathematica scientia,Series A, 2022, 42(2): 570-582. |
| [6] | Dandan Sun,Yingke Li,Zhidong Teng,Tailei Zhang. Analysis of the Stability for Measles Epidemic Model with Age-Structured [J]. Acta mathematica scientia,Series A, 2021, 41(6): 1950-1968. |
| [7] | Lixiang Feng,Defen Wang. Global Stability of an Epidemic Model with Quarantine and Incomplete Treatment [J]. Acta mathematica scientia,Series A, 2021, 41(4): 1235-1248. |
| [8] | Xiling Li,Fei Gao,Wenqin Li. Stability Analysis of Fractional-Order Hepatitis B Virus Infection Model With Immune Delay [J]. Acta mathematica scientia,Series A, 2021, 41(2): 562-576. |
| [9] | Jian Liu,Zhixin Zhang,Wei Jiang. Global Mittag-Leffler Stability of Fractional Order Nonlinear Impulsive Differential Systems with Time Delay [J]. Acta mathematica scientia,Series A, 2020, 40(4): 1053-1060. |
| [10] | Xiaojie Jing, Aimin Zhao, Guirong Liu. Global Stability of a Measles Epidemic Model with Partial Immunity and Environmental Transmission [J]. Acta mathematica scientia,Series A, 2019, 39(4): 909-917. |
| [11] | Haiyin Li. Hopf Bifurcation of Delayed Density-Dependent Predator-Prey Model [J]. Acta mathematica scientia,Series A, 2019, 39(2): 358-371. |
| [12] | Lifei Zheng,Jie Guo,Meihua Wu,Xiaorui Wang,Aying Wan. The Research on a Class of the Predator-Prey-Mutualist System with Delays [J]. Acta mathematica scientia,Series A, 2018, 38(5): 1001-1013. |
| [13] | Zhang Liping, Zhao Yu, Yuan Sanling. Threshold Dynamical Behaviors of a Stochastic SIS Epidemic Model with Nonlinear Incidence Rate [J]. Acta mathematica scientia,Series A, 2018, 38(1): 197-208. |
| [14] | Wei Aiju, Zhang Xinjian, Wang Junyi, Li Kezan. Dynamics Analysis of an Ebola Epidemic Model [J]. Acta mathematica scientia,Series A, 2017, 37(3): 577-592. |
| [15] | Zhang Shuwen. A Stochastic Predator-Prey System with Time Delays and Prey Dispersal [J]. Acta mathematica scientia,Series A, 2015, 35(3): 592-603. |
|