Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (2): 548-561.
Previous Articles Next Articles
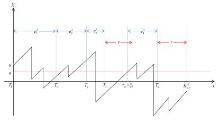
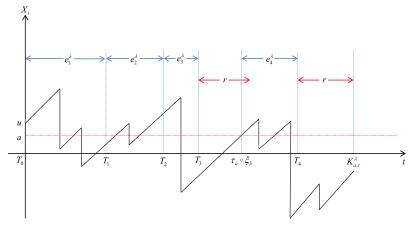
Parisian Ruin for Spectrally Negative Lévy Processes Under a Hybrid Observation Scheme
Xiaofeng Yang1,Hua Dong1,*( ),Hongshuai Dai2
),Hongshuai Dai2
- 1 School of Statistics, Qufu Normal University, Shandong Qufu 273165
2 School of Statistics, Shandong University of Finance and Economics, Jinan 250014
-
Received:2020-05-05Online:2021-04-26Published:2021-04-29 -
Contact:Hua Dong E-mail:sddh1978@126.com -
Supported by:the NSFC(11701319);the NSF of Shandong Province(ZR2019MA035);the NSF of Shandong Province(ZR2020MA035)
CLC Number:
- O211.6
Cite this article
Xiaofeng Yang,Hua Dong,Hongshuai Dai. Parisian Ruin for Spectrally Negative Lévy Processes Under a Hybrid Observation Scheme[J].Acta mathematica scientia,Series A, 2021, 41(2): 548-561.
share this article
| 1 |
Albrecher H , Cheung E C K , Thonhauser S . Randomized observation times for the compound Possion risk model: The discounted penalty function. Scand Actuar J, 2013, 2013 (6): 424- 452
doi: 10.1080/03461238.2011.624686 |
| 2 | Albrecher H , Ivanovs J , Zhou X . Exit identities for Lévy processes observed at Poisson arrival times. Bernoulli, 2016, 22 (3): 1364- 1382 |
| 3 |
Albrecher H , Kortschak D , Zhou X . Pricing of Parisian options for a jump-diffusion model with two-sided jumps. Appl Math Fina, 2012, 19 (2): 97- 129
doi: 10.1080/1350486X.2011.599976 |
| 4 |
Baurdoux E J , Pardo J C , Pérez J L , Renaud J-F . Gerber-Shiu distribution at Parisian ruin for Lévy insurance risk processes. J Appl Prob, 2016, 53 (2): 572- 584
doi: 10.1017/jpr.2016.21 |
| 5 | Bertoin J . Lévy Processes. Cambridge: Cambridge University Press, 1996 |
| 6 |
Czarna I , Palmowski Z . Ruin Probability with Parisian delay for a spectrally negative Lévy process. J Appl Prob, 2011, 48 (4): 984- 1002
doi: 10.1017/S0021900200008573 |
| 7 |
Dassios A , Wu S . On barrier strategy dividends with Parisian implementation delay for classical surplus process. Insurance Math Econ, 2009, 45 (2): 195- 202
doi: 10.1016/j.insmatheco.2009.05.013 |
| 8 |
Dong H , Yin C C , Dai H S . Spectrally negative Lévy risk model under Erlangized barrier strategy. J Comput Appl Math, 2019, 351, 101- 116
doi: 10.1016/j.cam.2018.11.001 |
| 9 |
Dong H , Zhou X . On a spectrally negative Lévy process with periodic dividends and capital injections. Statist Probab Lett, 2019, 155, 108589
doi: 10.1016/j.spl.2019.108589 |
| 10 | Kuznetsov A, Kyprianou A E, Rivero V. The Theory of Scale Functions for Spectrally Negative Lévy Processes//Cohen S, et al. Lévy Matters Π. Berlin Heidelberg: Springer, 2012: 97-186 |
| 11 | Kyprianou A E . Fluctuations of Lévy Processes with Applications. Introductory Lectures. Berlin Heidelberg: Springer, 2014 |
| 12 |
Landriault D , Renaud J-F , Zhou X . An insurance risk model with Parisian implementation delays. Methodol Comput Appl Probab, 2014, 16 (3): 583- 607
doi: 10.1007/s11009-012-9317-4 |
| 13 |
Landriault D , Renaud J-F , Zhou X . Occupation times of spectrally negative Lévy processes with applications. Stoch Process Appl, 2011, 121 (11): 2629- 2641
doi: 10.1016/j.spa.2011.07.008 |
| 14 |
Li B , Willmot G E , Wong J T Y . A temporal approach to the Parisian risk model. J Appl Prob, 2018, 55 (1): 302- 317
doi: 10.1017/jpr.2018.18 |
| 15 |
Li B , Zhou X . The joint Laplace transform for diffusion occupation times. Adv Appl Prob, 2013, 45 (4): 1049- 1067
doi: 10.1239/aap/1386857857 |
| 16 |
Li Y Q , Zhou X . On pre-exit joint occupation times for spectrally negative Lévy processes. Statist Probab Lett, 2014, 94, 48- 55
doi: 10.1016/j.spl.2014.06.023 |
| 17 |
Lkabous M A . A note on Parisian ruin under a hybrid observation scheme. Statist Probab Lett, 2019, 145, 147- 157
doi: 10.1016/j.spl.2018.09.013 |
| 18 |
Lkabous M A , Czarna I , Renaud J-F . Parisian ruin for a refracted Lévy process. Insurance Math Econ, 2017, 74, 153- 163
doi: 10.1016/j.insmatheco.2017.03.005 |
| 19 |
Lkabous M A , Renaud J-F . A unified approach to ruin probabilities with delays for spectrally negative Lévy processes. Scand Actuar J, 2019, 2019 (8): 711- 728
doi: 10.1080/03461238.2019.1598890 |
| 20 | Loeffen R L , Czarna I , Palmowski Z . Parisian ruin probability for spectrally negative Lévy processes. Bernoulli, 2013, 19 (2): 599- 609 |
| 21 |
Loeffen R L , Renaud J-F , Surya B A . Discounted penalty function at Parisian ruin for Lévy insurance risk process. Insurance Math Econ, 2018, 83, 190- 197
doi: 10.1016/j.insmatheco.2017.10.008 |
| 22 |
Loeffen R L , Renaud J-F , Zhou X . Occupation times of intervals until first passage times for spectrally negative Lévy processes. Stoch Process Appl, 2014, 124 (3): 1408- 1435
doi: 10.1016/j.spa.2013.11.005 |
| 23 | Wong J T Y , Cheung E C K . On the time value of Parisian ruin in (dual) renewal risk process with exponential jumps. Insurance Math Econ, 2015, 65 (2): 280- 290 |
| 24 |
张万路, 赵翔华. 折射Lévy风险过程的Parisian破产问题. 数学物理学报, 2019, 39A (1): 184- 199
doi: 10.3969/j.issn.1003-3998.2019.01.018 |
|
Zhang W L , Zhao X H . On the Parisian ruin probability in a refracted Lévy process. Acta Math Sci, 39A (1): 184- 199
doi: 10.3969/j.issn.1003-3998.2019.01.018 |
|
| 25 |
Zhao X H , Dong H , Dai H S . On spectrally positive Lévy risk processes with Parisian implementation delays in dividend payments. Statist Probab Lett, 2018, 140, 176- 184
doi: 10.1016/j.spl.2018.05.013 |
| [1] | Dong Deng,Yan Li. Traveling Waves in a Nonlocal Dispersal SIR Epidemic Model with Treatment [J]. Acta mathematica scientia,Series A, 2020, 40(1): 72-102. |
| [2] | Zhang Wanlu, Yin Xiaolong, Zhao Xianghua. On the Occupation Times in a Dual Delayed Sparre Andersen Risk Model [J]. Acta mathematica scientia,Series A, 2019, 39(4): 918-931. |
| [3] | Chun Wang. Stability of Some Fractional Systems and Laplace Transform [J]. Acta mathematica scientia,Series A, 2019, 39(1): 49-58. |
| [4] | Wanlu Zhang,Xianghua Zhao. On the Parisian Ruin Probability in a Refracted Lévy Process [J]. Acta mathematica scientia,Series A, 2019, 39(1): 184-199. |
| [5] | Guo Tingguang, Xu Zhiting. Existence of Traveling Waves in a Spatial Infectious Disease Model with Vaccination [J]. Acta mathematica scientia,Series A, 2017, 37(6): 1129-1147. |
| [6] | Deng Jiqin, Deng Ziming. Existence and Uniqueness of Solutions for Nonlocal Cauchy Problem for Fractional Evolution Equations [J]. Acta mathematica scientia,Series A, 2016, 36(6): 1157-1164. |
| [7] | ZHAO Xiang-Hua, YIN Chuan-Cun. A Jump-diffusion Risk Model with Dependence |between Claim Sizes and Claim Intervals [J]. Acta mathematica scientia,Series A, 2009, 29(6): 1657-1664. |
| [8] | LIU Juan, XU Jian-Cheng. Moments of the Discounted Dividends and Related Problems in a Markov-dependent Risk Model [J]. Acta mathematica scientia,Series A, 2009, 29(5): 1390-1397. |
| [9] |
Su Feng ;Peng Zhigang.
The Inverse Laplace Transform Theorem of Henstock-Kurzweil Integrable Function [J]. Acta mathematica scientia,Series A, 2007, 27(6): 1155-1163. |
|