Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (1): 166-177.
Previous Articles Next Articles
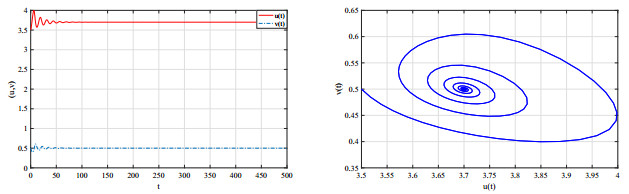
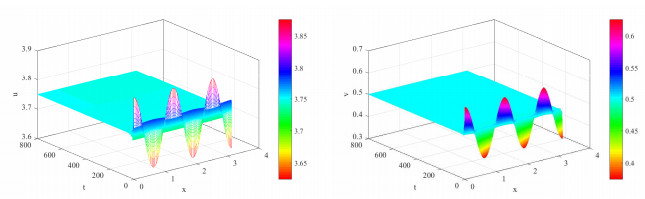
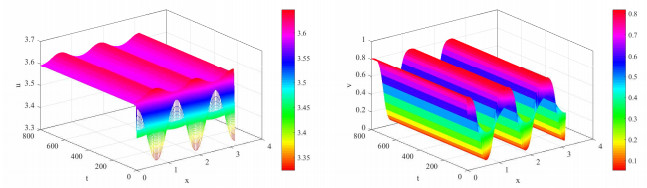
Hopf Bifurcation and Stability for an Autocatalytic Reversible Biochemical Reaction Model
- School of Arts and Sciences, Shaanxi University of Science and Technology, Xi'an 710021
-
Received:2020-01-09Online:2021-02-26Published:2021-01-29 -
Contact:Gaihui Guo E-mail:guogaihui@sust.edu.cn -
Supported by:the NSFC(61872227);the NSFC(61672021);the NSFC(11671243);the NSFC(11901370);the National Undergraduate Innovation and Entrepreneurship Training Program(201910708010)
CLC Number:
- O175.26
Cite this article
Gaihui Guo,Xiaohui Liu. Hopf Bifurcation and Stability for an Autocatalytic Reversible Biochemical Reaction Model[J].Acta mathematica scientia,Series A, 2021, 41(1): 166-177.
share this article
| 1 |
Jia Y F , Li Y , Wu J H . Qualitative analysis on positive steady-states for an autocatalytic reaction model in thermodynamics. Discrete and Continuous Dynamical Systems, 2017, 37 (9): 4785- 4813
doi: 10.3934/dcds.2017206 |
| 2 |
Gaspar V , Beck M T . Depressing the bistable behavior of the iodate-arsenous acid reaction in a continuous flow stirred tank reactor by the effect of chloride or bromide ions: A method for determination of rate constants. Journal of Physical Chemistry, 1986, 90 (23): 6303- 6305
doi: 10.1021/j100281a048 |
| 3 |
Finlayson A B , Merkin J H . Creation of spatial structure by an electric field applied to an ionic cubic autocatalator system. Journal of Engineering Mathematics, 2000, 38 (3): 279- 296
doi: 10.1023/A:1004799200173 |
| 4 |
Wei M H , Wu J H , He Y N . Steady-state solutions and stability for a cubic autocatalysis model. Communications on Pure and Applied Analysis, 2015, 14 (3): 1147- 1167
doi: 10.3934/cpaa.2015.14.1147 |
| 5 |
Guo G H , Li B F , Lin X L . Hopf bifurcation in spatially homogeneous and inhomogeneous autocatalysis models. Computers and Mathematics with Applications, 2014, 67 (1): 151- 163
doi: 10.1016/j.camwa.2013.08.014 |
| 6 |
Zhou J . Spatiotemporal pattern formation of a diffusive bimolecular model with autocatalysis and saturation law. Computers and Mathematics with Applications, 2013, 66 (10): 2003- 2018
doi: 10.1016/j.camwa.2013.08.022 |
| 7 |
Shi Q Y , Shi J P , Song Y L . Hopf bifurcation in a reaction-diffusion equation with distributed delay and Dirichlet boundary condition. Journal of Differential Equations, 2017, 263 (10): 6537- 6575
doi: 10.1016/j.jde.2017.07.024 |
| 8 |
李海银. 密度制约且具有时滞捕食-被捕食模型的Hopf分支. 数学物理学报, 2019, 39A (2): 358- 371
doi: 10.3969/j.issn.1003-3998.2019.02.015 |
|
Li H Y . Hopf bifurcation of delayed density-dependent predator-prey model. Acta Mathematica Scientia, 2019, 39A (2): 358- 371
doi: 10.3969/j.issn.1003-3998.2019.02.015 |
|
| 9 |
Yi F Q , Liu J X , Wei J J . Spatiotemporal pattern formation and multiple bifurcations in a diffusive bimolecular model. Nonlinear Analysis: Real World Applications, 2010, 11 (5): 3770- 3781
doi: 10.1016/j.nonrwa.2010.02.007 |
| 10 |
周军. 一类具有自动催化作用和饱和定律的双分子模型的图灵不稳定性和霍普夫分歧. 数学物理学报, 2017, 37A (2): 366- 373
doi: 10.3969/j.issn.1003-3998.2017.02.015 |
|
Zhou J . Turing instability and Hopf bifurcation of a bimolecular model with autocatalysis and saturation law. Acta Mathematica Scientia, 2017, 37A (2): 366- 373
doi: 10.3969/j.issn.1003-3998.2017.02.015 |
|
| 11 | Wiggins S . Introduction to Applied Nonlinear Dynamical Systems and Chaos. New York: Springer, 1991: 1- 280 |
| 12 | Yi F Q , Wei J J , Shi J P . Bifurcation and spatiotemporal patterns in a homogeneous diffusive predator-prey system. Journal of Differential Equations, 2009, 246 (5): 1944- 1977 |
| 13 | Hassard B D , Kazarinoff N D , Wan Y H . Theory and Application of Hopf bifurcation. Cambridge: Cambridge University Press, 1981 |
| [1] | Du Dandan, Zhu Yucan. Stabilities of K-Frames and Tight K-Frames Under the Operator Perturbation [J]. Acta mathematica scientia,Series A, 2021, 41(1): 29-38. |
| [2] | Zerong He,Zhiqiang Zhang,Yang Wang. Stability of a Class of Nonlinear Hierarchical Age-Dependent Population Model [J]. Acta mathematica scientia,Series A, 2020, 40(6): 1712-1722. |
| [3] | Wei Li,Pengzhan Huang. A Viscosity-Splitting Finite Element Method for the Fluid-Fluid Interaction Problem [J]. Acta mathematica scientia,Series A, 2020, 40(5): 1362-1380. |
| [4] | Yonghui Zhou. Global Stability of the Nonmonotone Critical Traveling Waves for Reaction Diffusion Equations [J]. Acta mathematica scientia,Series A, 2020, 40(4): 983-992. |
| [5] | Jian Liu,Zhixin Zhang,Wei Jiang. Global Mittag-Leffler Stability of Fractional Order Nonlinear Impulsive Differential Systems with Time Delay [J]. Acta mathematica scientia,Series A, 2020, 40(4): 1053-1060. |
| [6] | Xingshou Huang,Ricai Luo,Wusheng Wang. Stability Analysis for a Class Neural Network with Proportional Delay Based on the Gronwall Integral Inequality [J]. Acta mathematica scientia,Series A, 2020, 40(3): 824-832. |
| [7] | Chun Wang,Tianzhou Xu. Hyers-Ulam-Rassias Stability of a Mixed Type Cubic-Quartic Functional Equation in 2-Banach Spaces [J]. Acta mathematica scientia,Series A, 2020, 40(2): 352-368. |
| [8] | Menglan Liao,Bin Guo. Asymptotic Stability of Weak Solutions to Wave Equation with Variable Exponents and Strong Damping Term [J]. Acta mathematica scientia,Series A, 2020, 40(1): 146-155. |
| [9] | Ru Wang,Zhonghua Zhang,Yeling Liu. Dynamical Properties of a Discontinuous Models with Signal Molecules Regulation [J]. Acta mathematica scientia,Series A, 2019, 39(6): 1545-1554. |
| [10] | Changxu Shao,Shutang Liu. Fractal Feature and Control of Three-Species Predator-Prey Model [J]. Acta mathematica scientia,Series A, 2019, 39(4): 951-962. |
| [11] | Chao Yang,Runjie Li. Existence and Stability of Periodic Solution for a Lasota-Wazewska Model with Discontinuous Harvesting [J]. Acta mathematica scientia,Series A, 2019, 39(4): 785-796. |
| [12] | Qinghua Zhou,Li Wan,Jie Liu. Global Attracting Set for Neutral Type Hopfield Neural Networks with Time-Varying Delays [J]. Acta mathematica scientia,Series A, 2019, 39(4): 823-831. |
| [13] | Yanhua Shi,Yadong Zhang,Fenling Wang,Yanmin Zhao,Pingli Wang. High Accuracy Analysis of Linear Triangular Element for Time Fractional Diffusion Equations [J]. Acta mathematica scientia,Series A, 2019, 39(4): 839-850. |
| [14] | Xiaojie Jing, Aimin Zhao, Guirong Liu. Global Stability of a Measles Epidemic Model with Partial Immunity and Environmental Transmission [J]. Acta mathematica scientia,Series A, 2019, 39(4): 909-917. |
| [15] | Gengen Zhang,Wansheng Wang,Aiguo Xiao. Asymptotic Estimation of the Trapezoidal Method for a Class of Neutral Differential Equation with Variable Delay [J]. Acta mathematica scientia,Series A, 2019, 39(3): 560-569. |
|