Acta mathematica scientia,Series A ›› 2021, Vol. 41 ›› Issue (1): 15-28.
Previous Articles Next Articles
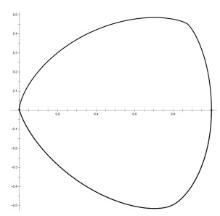
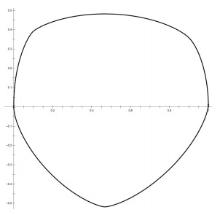
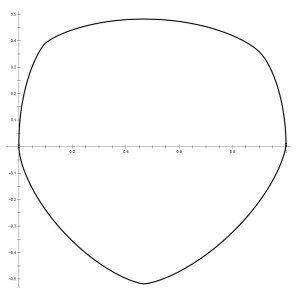
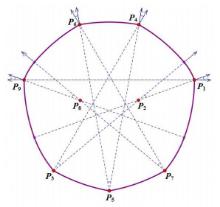
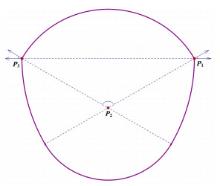
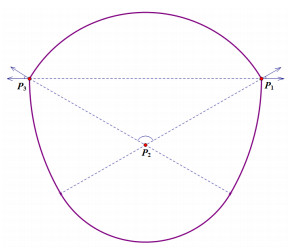
Construction of the Planar Bodies with Constant Width
- School of Mathematical Sciences, Huaibei Normal University, Anhui Huaibei 235000
CLC Number:
- O174.13