一类分数阶Langevin方程预估校正算法的数值分析

Abstract

Abstract:

This paper extends the Langevin equation on the fractional order to make it time-memory, and a class of fractional-order Langevin equation is solved numerically using the predictor-corrector method. Firstly, the estimated value is obtained by R0 algorithm, then the estimated value is substituted into the R2 algorithm to correct the numerical solution. Finally, the numerical solution of a class of fractional-order Langevin equation by predictor-corrector method is obtained. The error analysis proves that under the condition of $0 < \alpha < 1$ of the equation, the result of predictor-corrector method is $(1+\alpha)$ order convergence. Numerical experiments also show that the numerical solution of the predictor-corrector method is convergent under different values of $\alpha$ and step size $h$.

Key words: Fractional Langevin equation, Predictor-Corrector method, Error analysis, Numerical experiments

CLC Number:

O211.63

Linjuan Pu,Xiaozhong Yang,Shuzhen Sun. Numerical Analysis of a Class of Fractional Langevin Equation by Predictor-Corrector Method[J].Acta mathematica scientia,Series A, 2020, 40(4): 1018-1028.

Trendmd

Figures/Tables 8

References 22

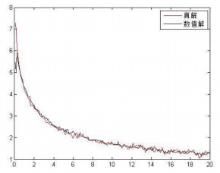
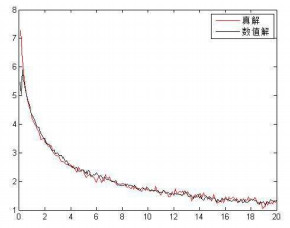
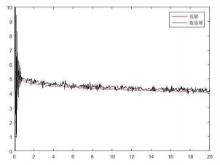
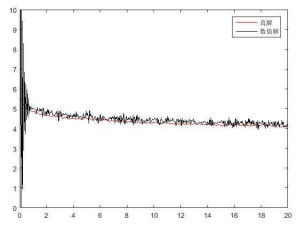
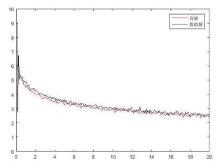
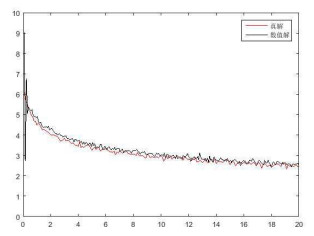
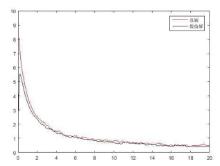
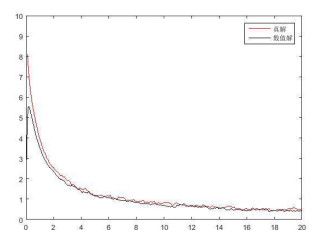
1	包景东. 反常统计动力学导论. 北京: 科学出版社, 2012
	Bao J D . Introduction to Anomalous Statistical Dynamics. Beijing: Science Press, 2012
2	Sergio P , West B J . Fractional Langevin model of memory in financial markets. Physical Review E, 2002, 66 (2): 046118
3	West B J , Sergio P . Fractional Langevin model of memory in financial time series. Physical Review E, 2002, 65 (2): 037106
4	Fa K S . Generalized Langevin equation with fractional derivative and long-time correlation function. Physical Review E, 2006, 73 (1): 061104
5	Fa K S . Fractional Langevin equation and Riemann-Liouville fractional derivative. The European Physical Journal E-Soft Matter, 2007, 24 (2): 139- 143 doi: 10.1140/epje/i2007-10224-2
6	Jeon J H , Metzler R . Fractional brownian motion and motion governed by the fractional Langevin equation in confined geometries. Phys Rev E, 2010, 81 (1): 021103
7	Lutz E . Fractional Langevin equation. Phys Rev E, 2001, 64, 051106 doi: 10.1103/PhysRevE.64.051106
8	Camargo R F , Chiacchio A O , Charnet R , et al. Solution of the fractional Langevin equation and the Mittag-Leffler functions. Journal of Mathematical Physics, 2009, 50 (6): 064606
9	Bhrawy A H , Alghamdi M A . A shifted Jacobi-Gauss-Lobatto collocation method for solving nonlinear fractional Langevin equation involving two fractional orders in different intervals. Boundary Value Problems, 2012, 2012 (1): 62
10	Diethelm K , Ford N J , Freed A D . A predictor-corrector approach for the numerical solution of fractional differential equations. Nonlinear Dynamics, 2002, 29 (4): 3- 22
11	Bruti-Liberati N , Platen E . Strong predictor-corrector Euler methods for stochastic differential equations. Stochastics and Dynamics, 2008, 08 (3): 561- 581
12	Ye J , Li H , Xiao L . Strong predictor-corrector Euler-Maruyama methods for stochastic differential equations with Markovian switching. Journal of Computational and Applied Mathematics, 2011, 237 (1): 5- 17
13	Niu Y , Zhang C . Almost sure and moment exponential stability of predictor-corrector methods for stochastic differential equations. Journal of Systems Science and Complexity, 2012, 25 (4): 736- 743
14	Niu Y , Zhang C , Burrage K . Strong predictor-corrector approximation for stochastic delay differential equations. Mathematica Numerica Sinica, 2015, 33 (6): 587- 605
15	Xiao F , Wang P . Strong predictor-corrector methods for stochastic pantograph equations. Mathematica Numerica Sinica, 2016, 34 (1): 1- 11
16	Khaliq A , Biala T A , Alzahrani S S , et al. Linearly implicit predictor-corrector methods for space-fractional reaction-diffusion equations with non-smooth initial data. Computers and Mathematics with Applications, 2018, 75 (8): 2629- 2657 doi: 10.1016/j.camwa.2017.12.033
17	Diethelm K , Ford N J . Analysis of fractional differential equations. Journal of Mathematical Analysis and Applications, 2002, 265 (2): 229- 248
18	郭柏灵, 蒲学科, 黄凤辉. 分数阶偏微分方程及其数值解. 北京: 科学出版社, 2011
	Guo B L , Pu X K , Huang F H . Fractional Partial Differential Equations and Their Numerical Solutions. Beijing: Science Press, 2011
19	Diethelm K , Ford N J , Freed A D . Detailed error analysis for a fractional adams method. Numerical Algorithms, 2004, 36 (1): 31- 52
20	赵雅迪, 吴立飞, 杨晓忠, 孙淑珍. 时间分数阶慢扩散方程的一类有效差分方法. 数学物理学报, 2018, 38A (6): 1122- 1134 doi: 10.3969/j.issn.1003-3998.2018.06.009
	Zhao Y D , Wu L F , Yang X Z , Sun S Z . A kind of efficient difference method for the time fractional sub-diffusion equation. Acta Math Sci, 2018, 38A (6): 1122- 1134 doi: 10.3969/j.issn.1003-3998.2018.06.009
21	Biagini F , Hu Y , Oksendal , et al. Stochastic calculus for fractional brownian motion and applications. Siam Journal on Control and Optimization, 2008, 38 (2): 582- 612
22	薛定宇, 陈阳泉. 高等应用数学问题的MATLAB求解(第三版). 北京: 清华大学出版社, 2013
	Xue D Y , Chen Y Q . Advanced Applied Mathematical Problem Solutions with MATLAB (Third Edition). Beijing: Tsinghua University Press, 2013

$\alpha$	$h$	$e(h)$	$\gamma$	$\alpha$	$h$	$e(h)$	$\gamma$
	0.005	0.0034			0.005	0.0010
	0.008	0.0105			0.008	0.0028
0.1	0.01	0.0117	1.0732	0.3	0.01	0.0026	1.2005
	0.05	0.0638			0.05	0.0635
	0.1	0.0941			0.1	0.018
	0.005	0.0013			0.005	0.0012
	0.008	0.0013			0.008	0.0022
0.5	0.01	0.0032	1.3476	0.7	0.01	0.0041	1.5506
	0.05	0.0364			0.05	0.0578
	0.1	0.0462			0.1	0.1037
	0.005	0.0006
	0.008	0.0007
0.9	0.01	0.0015	1.8435
	0.05	0.0478
	0.1	0.0850

[1]	Yuji Liu. Solvability of BVPs for Impulsive Fractional Langevin Type Equations Involving Three Riemann-Liouville Fractional Derivatives [J]. Acta mathematica scientia,Series A, 2020, 40(1): 103-131.
[2]	Yadi Zhao, Lifei Wu, Xiaozhong Yang, Shuzhen Sun. A Kind of Efficient Difference Method for the Time Fractional Sub-Diffusion Equation [J]. Acta mathematica scientia,Series A, 2018, 38(6): 1122-1134.
[3]	WEN Juan, HE Yin-Nian, WANG Xue-Min, HUO Mi-Hui. TWO-LEVEL MULTISCALE FINITE ELEMENT METHODS FOR THE STEADY NAVIER-STOKES PROBLEM [J]. Acta mathematica scientia,Series A, 2014, 34(3): 960-972.

Numerical Analysis of a Class of Fractional Langevin Equation by Predictor-Corrector Method

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 8

References 22

Related Articles 3

Metrics

Comments

Recommended 0