一类非线性年龄等级结构种群模型的数值解法

Abstract

Abstract:

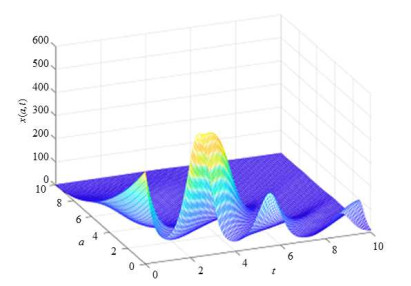
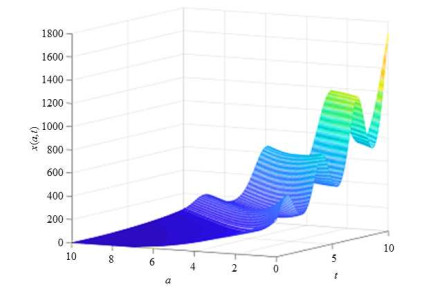
Based upon the assumption that the young individuals are more competitive than the old ones within a species, a class of nonlinear hierarchical age-structured population model is established in a form of IBVP of integro-partial differential equations. We propose an algorithm for the solutions to the model, analyze its convergence, and make some numerical experiments.

Key words: Hierarchy of age, Population model, Numerical method, Discrete Gronwall's inequality, Convergence

CLC Number:

O211.4

Zerong He,Zhiqiang Zhang,Zheyong Qiu. Numerical Method of a Nonlinear Hierarchical Age-Structured Population Model[J].Acta mathematica scientia,Series A, 2020, 40(2): 515-526.

Trendmd

Figures/Tables 2

References 23

1	López-Marcos J C . An upwind scheme for a nonlinear hyperbolic integro-differential equation with integral boundary condition. Computers Math Applic, 1991, 22 (11): 15- 28 doi: 10.1016/0898-1221(91)90030-8
2	Fairweather G , López-Marcos J C . A box method for a nonlinear equation of population dynamics. IMA Journal of Numerical Analysis, 1991, 11, 525- 538 doi: 10.1093/imanum/11.4.525
3	Sulsky D . Numerical solution of structured population models Ⅰ. Age structure. J Math Biol, 1993, 31, 817- 839 doi: 10.1007/BF00168048
4	Guo B Z , Sun B . Numerical solution to the optimal birth feedback control of a population dynamics:viscosity solution approach. Opitmal Control Applications and Methods, 2005, 26, 229- 254 doi: 10.1002/oca.759
5	Abia L M , Angulo O , López-Marcos J C . Age-structured population models and their numerical solution. Ecological Modelling, 2005, 188, 112- 136 doi: 10.1016/j.ecolmodel.2005.05.007
6	Kim M Y , Selenge T . Age-time continuous Galerkin methods for a model of population dynamics. Journal of Computational and Applied Mathematics, 2009, 223, 659- 671 doi: 10.1016/j.cam.2008.02.004
7	Angulo O , López-Marcos J C , López-Marcos M A , Milner F A . A numerical method for nonlinear age-structured population models with finite maximum age. J Math Anal Appl, 2010, 361 (1): 150- 160
8	Iannelli M, Milner F. The Basic Approach to Age-Structured Population Dynamics:Models, Methods and Numerics//Mackey M, Stevens A. Lecture Notes on Mathematical Modelling in the Life Sciences. Dordrecht:Springer, 2017:89-122
9	Banks H T . Computational techniques for inverse problems in size structured stochastic population models. Lecture Notes in Control and Information, 1989, 114, 3- 10
10	Ito K , Kappel F , Peichl G . A fully discretized approximation scheme for size-structured population models. SIAM J Numer Anal, 1991, 28 (4): 923- 954 doi: 10.1137/0728050
11	Sulsky D . Numerical solution of structured population models Ⅱ. Mass structure. J Math Biol, 1994, 32, 491- 514 doi: 10.1007/BF00160170
12	Angulo O , López-Marcos J C . Numerical scheme for size-structured population equations. Mathematical Biosciences, 1999, 157, 169- 188 doi: 10.1016/S0025-5564(98)10081-0
13	Angulo O , López-Marcos J C . Numerical integration of autonomous and non-autonomous nonlinear size-structured population models. Mathematical Biosciences, 2002, 177, 39- 71
14	Avbia L M , Angulo O , López-Marcos J C . Size-structured population dynamics models and their numerical solutions. Discrete and Continuous Dynamical Systems-Series B, 2004, 4 (4): 1203- 1222 doi: 10.3934/dcdsb.2004.4.1203
15	Angulo O , Durán A , López-Marcos J C . Numerical study of size-structured population models:A case of Gambussia affinis. C R Biologies, 2005, 328, 387- 402 doi: 10.1016/j.crvi.2004.11.007
16	Angulo O , López-Marcos J C , López-Marcos M A . Numerical approximation of singular asymptotic states for a size-structured population model with dynamical resource. Mathematical and Computer Modelling, 2011, 54, 1693- 1698 doi: 10.1016/j.mcm.2010.12.006
17	Ackleh A S , Ito K . An implicit finite difference scheme for the nonlinear size-structured population model. Numerical Functional Analysis and Optimization, 1997, 18 (9/10): 865- 884
18	何泽荣, 杨立志. 具有尺度结构和双加权的种群模型:稳定性与最优收获. 数学物理学报, 2016, 36A (3): 584- 600
	He Z , Yang L . A weighted population model with size-structure:Stability and optimal harvesting. Acta Mathematica Scientia, 2016, 36A (3): 581- 600
19	Ackleh A S , Deng K , Hu S . A quasilinear hierarchical size-structured model:well-posedness and approximation. Appl Math Optim, 2005, 51, 35- 59 doi: 10.1007/s00245-004-0806-2
20	Shen J , Shu C W , Zhang M . A high order WENO scheme for a hierarchical size-structured population model. J Sci Comput, 2007, 33, 279- 291 doi: 10.1007/s10915-007-9152-x
21	Ackleh A S , Deng K , Thibodeaux J J . A monotone approximation for a size-structured population model with a generalized environment. Journal of Biological Dynamics, 2007, 1 (4): 305- 319 doi: 10.1080/17513750701605564
22	何泽荣, 张智强, 王淑平. 一类非线性等级结构种群控制模型解的适定性. 系统科学与数学, 2019, 39 (8): 1201- 1211
	He Z , Zhang Z , Wang S . The well-posedness of a nonlinear hierarchical age-structured population control model. J Sys Sci & Math Scis, 2019, 39 (8): 1201- 1211
23	López-Marcos J C , Sanz-Serna J M . Stability and convergence in numerical analysis Ⅲ:Linear investigation of nonlinear stability. IMA Journal of Numerical Analysis, 1988, 8 (1): 71- 84 doi: 10.1093/imanum/8.1.71

[1]	Gang Cai. Viscosity Implicit Algorithms for a Variational Inequality Problem and Fixed Point Problem in Hilbert Spaces [J]. Acta mathematica scientia,Series A, 2020, 40(2): 395-407.
[2]	Yuxian Hu. A Double Projection Method for Solving Variational Inequalities [J]. Acta mathematica scientia,Series A, 2019, 39(6): 1492-1498.
[3]	Jianhua Huang,Zaiyun Zhang,Yong Chen. The Moderate Deviation Principle for Stochastic 3D LANS-α Model Driven by Multiplicative Lévy Noise [J]. Acta mathematica scientia,Series A, 2019, 39(6): 1532-1544.
[4]	Juan Wang,Jie Zhao. Homogenization of the Neumann Boundary Value Problem: The Sharper W^{1, p} Estimate [J]. Acta mathematica scientia,Series A, 2019, 39(5): 1115-1124.
[5]	Conggang Liang,Xiaoxia Yang,Dongyang Shi. Convergence Analysis of Wilson Element for Parabolic Integro-Differential Equation [J]. Acta mathematica scientia,Series A, 2019, 39(5): 1158-1169.
[6]	Qian Zhang,Guanghui Cai. Complete Convergence and Complete Moment Convergence for WOD Random Variables Sequences [J]. Acta mathematica scientia,Series A, 2019, 39(5): 1183-1191.
[7]	Yanhua Shi,Yadong Zhang,Fenling Wang,Yanmin Zhao,Pingli Wang. High Accuracy Analysis of Linear Triangular Element for Time Fractional Diffusion Equations [J]. Acta mathematica scientia,Series A, 2019, 39(4): 839-850.
[8]	Xiaoling Qiu,Wensheng Jia. An Approximation Theorem of Variational Inequalities Under Bounded Rationality [J]. Acta mathematica scientia,Series A, 2019, 39(4): 730-737.
[9]	Zhihua Zhang,Pingyan Chen. Complete Convergence for Weighted Sums for $φ$-Mixing Random Variables [J]. Acta mathematica scientia,Series A, 2018, 38(6): 1095-1102.
[10]	Dehua Qiu, Juan Xiao. Complete Moment Convergence for Sung's Type Weighted Sums Under END Setup [J]. Acta mathematica scientia,Series A, 2018, 38(6): 1103-1111.
[11]	Yadi Zhao, Lifei Wu, Xiaozhong Yang, Shuzhen Sun. A Kind of Efficient Difference Method for the Time Fractional Sub-Diffusion Equation [J]. Acta mathematica scientia,Series A, 2018, 38(6): 1122-1134.
[12]	Zhifeng Zhu,Shaoyi Zhang. Study on the Convergence of Nonhomogeneous Markov Chains with Probability Distance [J]. Acta mathematica scientia,Series A, 2018, 38(5): 963-969.
[13]	Yang Xuan, Ruan Xiaoe, Wang Peng. Convergence Analysis of Iterative Learning Control for Linear Continuous-Time Switched Systems with Arbitrary Time-Driven Switching Rules [J]. Acta mathematica scientia,Series A, 2018, 38(3): 599-612.
[14]	Chen Hongbin, Gan Siqing, Xu Da, Peng Yulong. A Formally Second-Order BDF Compact ADI Difference Scheme for the Two-Dimensional Fractional Evolution Equation [J]. Acta mathematica scientia,Series A, 2017, 37(5): 976-992.
[15]	Fu Zongkui, Wu Qunying. A General Result of Precise Asymptotics in Complete Moment Convergence for ρ-mixing Sequence [J]. Acta mathematica scientia,Series A, 2017, 37(3): 544-552.

Numerical Method of a Nonlinear Hierarchical Age-Structured Population Model

RichHTML

PDF (PC)

Abstract

Cite this article

share this article

Figures/Tables 2

References 23

Related Articles 15

Metrics

Comments

Recommended 0