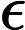[1] Akbari F, Ghaznavi M, Khorram E. A revised Pascoletti-Serafini scalarization method form multiobjective optimization problems. J Optim Theory Appl, 2018, 178(2): 560-590
[2] Annamdas K K, Rao S S. Multi-objective optimization of engineering systems using game theory and particle swarm optimization. Eng Optim, 2009, 41(8): 737-752
[3] Branke J, Deb K, Miettinen K, Sowiski R.Multiobjective Optimization Interactive and Evolutionary Approaches. Berlin: Springer, 2008
[4] Burachik R S, Kaya C Y, Rizvi M M. A new scalarization technique and new algorithms to generate Pareto fronts. SIAM J Optim, 2017, 27(2): 1010-1034
[5] Burachik R S, Kaya C Y, Rizvi M M. A new scalarization technique to approximate Pareto fronts of problems with disconnected feasible sets. J Optim Theory Appl, 2014, 162(2): 428-446
[6] Chankong V, Haimes, Y Y.Multiobjective Decision Making: Theory and Methodology. Amsterdam: North Holland, 1983
[7] Chuong T D, Kim D S. Approximate solutions of multiobjective optimization problems. Positivity, 2016, 20: 187-207
[8] Coello Coello C A, Lamont G B, Van Veldhuizen D A. Evolutionary Algorithms for Solving Multiobjective Problems. Genetic Algorithms and Evolutionary Computation Series. New York: Springer, 2007
[9] Deb K.Multi-Objective Optimization Using Evolutionary Algorithms, Wiley-Interscience Series in Systems and Optimization. Chichester: Wiley, 2001
[10] Doerner K, Gutjahr W J, Hartl R F, Strauss C, Stummer C. Pareto ant colony optimization: A metaheuristic approach to multiobjective portfolio selection. Ann Oper Res, 2004, 131: 79-99
[11] Ehrgott M. Multicriteria Optimization. Berlin: Springer, 2005
[12] Ehrgott M, Ruzika S. Improved 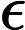 -constraint method for multiobjective programming. J Optim Theory Appl, 2008, 138: 375-396 -constraint method for multiobjective programming. J Optim Theory Appl, 2008, 138: 375-396
[13] Eichfelder G.Adaptive Scalarization Methods in Multiobjective Optimization. Berlin: Springer, 2008
[14] Eichfelder G. An adaptive scalarization method in multi-objective optimization. SIAM J Optim, 2009, 19: 1694-1718
[15] Eichfelder G. Scalarizations for adaptively solving multi-objective optimization problems. Comput Optim Appl, 2009, 44: 249-273
[16] Engau A, Wiecek M M. Generating 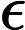 -efficient solutions in multiobjective programming. Eur J Oper Res, 2007, 177(3): 1566-1579 -efficient solutions in multiobjective programming. Eur J Oper Res, 2007, 177(3): 1566-1579
[17] Geoffrion A. Proper efficiency and the theory of vector maximization. J Math Anal Appl, 1968, 22(3): 618-630
[18] Ghaznavi M, Azizi Z. An algorithm for approximating nondominated points of convex multiobjective optimization problems. Bull Iranian Math Soc, 2017, 43(5): 1399-1415
[19] Ghaznavi M, Akbari F, Khorram E. Optimality conditions via a unified direction approach for (approximate) efficiency in multiobjective optimization. Optim Methods Softw, 2021, 36(2/3): 627-652
[20] Golestani M, Nobakhtian S. Nonsmooth multiobjective programming: strong Kuhn-Tucker conditions. Positivity, 2013, 17: 711-732
[21] Hillermeier C, Jahn J. Multiobjective optimization: survey of methods and industrial applications. Surv Math Ind, 2005, 11: 1-42
[22] Hoseinpoor N, Ghaznavi M. The modified objective-constraint scalarization approach for multiobjective optimization problems. Hacet J Math Stat, 2022, 51(5): 1403-1418
[23] Huband S, Hingston P, Barone L, While L. A review of multi-objective test problems and a scalable test problem toolkit. IEEE Trans Evol Comput, 2006, 10(5): 477-506
[24] Kasimbeyli R, Ozturk Z K, Kasimbeyli N, et al.Comparison of some scalarization methods in multiobjective optimization. Bull Malays Math Sci Soc, 2019, 42(5): 1875-1905
[25] Kuhn H, Tucker A.Nonlinear programming//Neyman J. Proceedings of the Second Berkeley Symposium on Mathematical Statistics and Probability. Berkeley, California: University of California Press, 1951: 481-492
[26] Marler R T, Arora J S. Survey of multi-objective optimization methods for engineering. Struct Multidiscip Optim, 2004, 26(6): 369-395
[27] Mueller-Gritschneder D, Graeb H, Schlichtmann U. A successive approach to compute the bounded Pareto front of practical multiobjective optimization problems. SIAM J Optim, 2009, 20: 915-934
[28] Pascoletti A, Serafini P. Scalarizing vector optimization problems. J Optim Theory Appl, 1984, 42: 499-524
[29] Rizvi M M.New optimality conditions for non-linear multiobjective optimization problems and new scalarization techniques for constructing pathological Pareto fronts[D]. South Australia: University of South Australia, 2013
[30] Sangiorgio V, Parisi F. A multicriteria approach for risk assessment of Covid-19 in urban district lockdown. Saf Sci, 2020, 130: 104862
[31] Steuer R E, Na P. Multiple criteria decision making combined with finance: a categorized bibliographic study. Eur J Oper Res, 2003, 150(3): 496-515
[32] Youness Mamdouh E A, Elbrolosy E. Extensions of proper efficiency in complex space. Math Methods Appl Sci, 2017, 41(15): 5792-5800 |