Processing math: 100%

阶跃初值条件解的完全分类: 流体力学中广义 Gardner 方程的分析与数值验证
张岩,郝惠琴,郭睿

The Complete Classification of Solutions to the Step Initial Condition: Analysis and Numerical Verification for the Generalized Gardner Equation in Fluid Mechanics
Yan Zhang,Huiqin Hao,Rui Guo
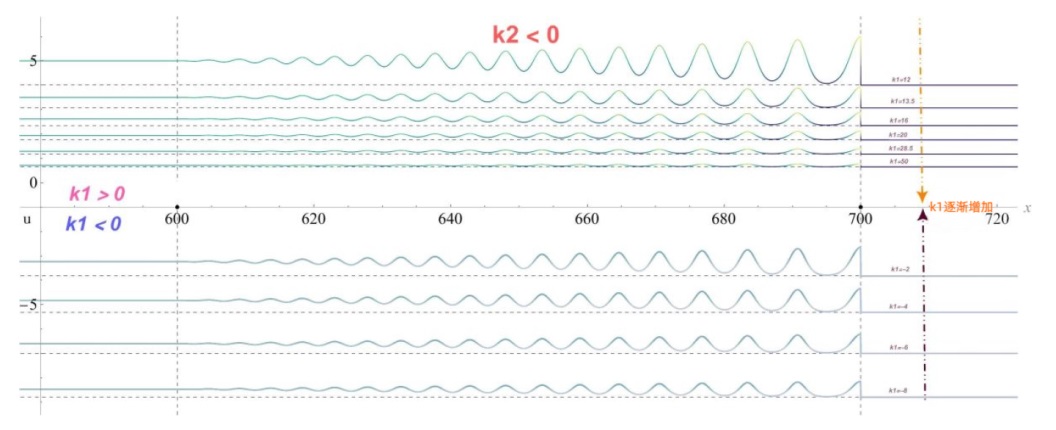
图42 当 k1 变化时, t=20 时刻区域 1 内正向色散激波的演化行为. 当 k1>0 时, 从上到下的参数如下: u−=5, u+=4; u−=7/2, u+=14(27−√217); u−=8−√29, u+=8−4√2; u−=10−√65, u+=10−2√17; u−=14(57−√2689), u+=14(57−√2737); u−=25−√590, u+=25−√593 并且 k2=−1, d=1. 当 k1<0 时, u−=−1−√5, u+=−1−2√2; u−=−2−2√2, u+=−2−√11; u−=−3−√13, u+=−7; u−=−4−2√5, u+=−4−√23 并且 k2=−1, d=40.